Learn how to integrate the square root of x step-by-step. We start by identifying the function to integrate and rewriting the square root as a power. We then apply the power rule for integration and simplify the resulting expression. Finally, we convert the result back to root form. The solution to the integral of the square root of x is (2/3)(√x)^3 + C.
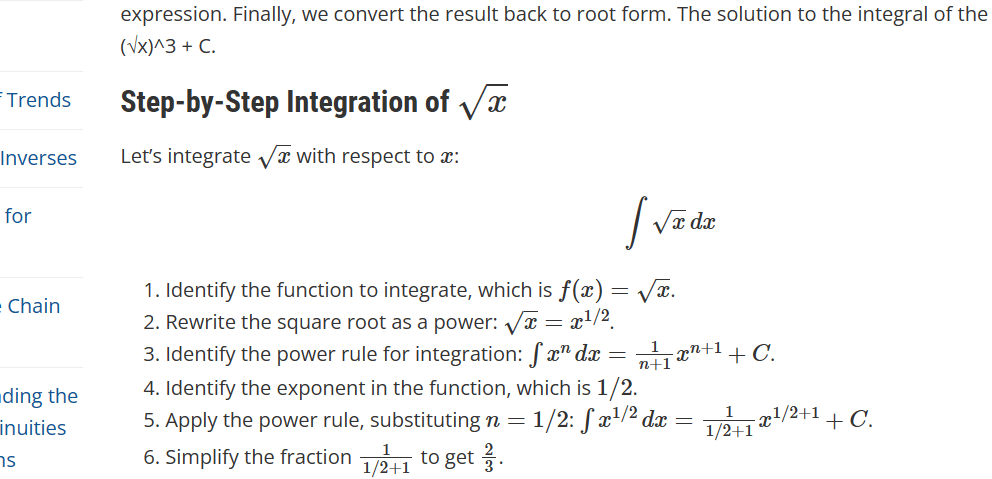
Step-by-Step Integration of \(\sqrt{x}\)
Let’s integrate \(\sqrt{x}\) with respect to \(x\):
\[ \int \sqrt{x} \, dx \]
- Identify the function to integrate, which is \(f(x) = \sqrt{x}\).
- Rewrite the square root as a power: \(\sqrt{x} = x^{1/2}\).
- Identify the power rule for integration: \(\int x^n \, dx = \frac{1}{n+1}x^{n+1} + C\).
- Identify the exponent in the function, which is \(1/2\).
- Apply the power rule, substituting \(n = 1/2\): \(\int x^{1/2} \, dx = \frac{1}{1/2+1}x^{1/2+1} + C\).
- Simplify the fraction \(\frac{1}{1/2+1}\) to get \(\frac{2}{3}\).
- Simplify the exponent \(1/2+1\) to get \(3/2\), so the antiderivative is \(\frac{2}{3}x^{3/2} + C\).
- Convert the result to root form. The exponent \(3/2\) means we take the square root of \(x\) and then cube the result, which gives \((\sqrt{x})^3\). So, the antiderivative can also be written as \(\frac{2}{3}(\sqrt{x})^3 + C\).
Solution:
The antiderivative of \(\sqrt{x}\) is \(\frac{2}{3}(\sqrt{x})^3 + C\).