Are you looking to understand the intricacies of finding partial derivatives of exponential functions with negative exponents? In this comprehensive guide, we break down the process of differentiating the function e^(-xyz) with respect to each variable x, y, and z. Through detailed step-by-step examples, we explore how to apply the chain rule and other differentiation techniques to find the partial derivatives of this specific exponential function. Whether you’re a student, educator, or math enthusiast, this guide provides valuable insights to enhance your understanding of multivariable calculus.
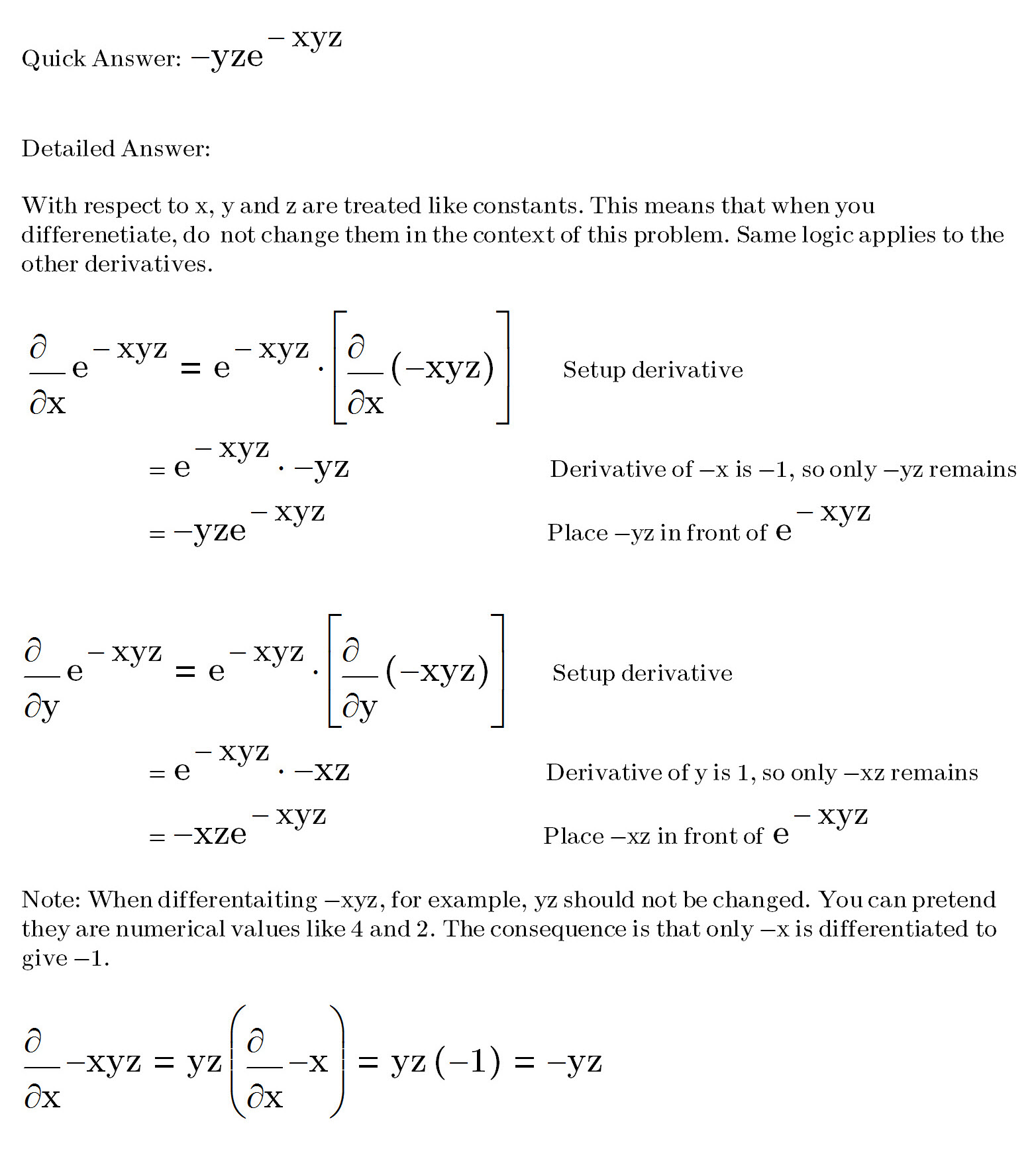
Finding the Partial Derivative of
| Start with the given function. | |
| Indicate that we are taking the derivative with respect to |
|
| Apply the chain rule by multiplying the derivative of the inside function |
|
| Differentiate |
|
| Since we are taking the partial derivative with respect to |
|
| Write the final expression for the partial derivative of |
This step-by-step guide offers a detailed and clear explanation of finding the partial derivative of the function
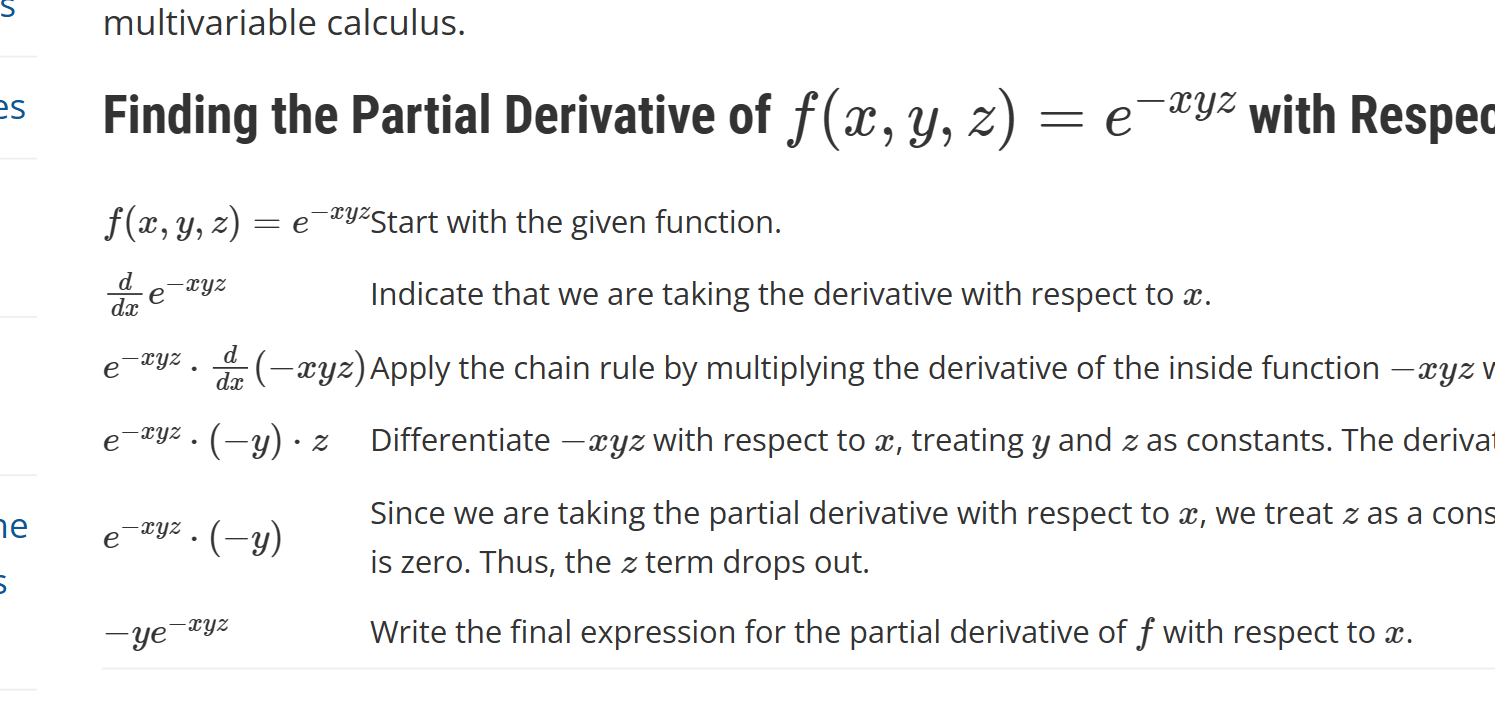
Finding the Partial Derivative of
Understanding the process of finding partial derivatives is essential in multivariable calculus. In this guide, we focus on finding the partial derivative of the function
| Indicate that we are taking the derivative with respect to |
|
| Apply the chain rule by multiplying the derivative of the inside function |
|
| Differentiate |
|
| Since we are taking the partial derivative with respect to |
|
| Write the final expression for the partial derivative of |
This step-by-step guide provides a clear and comprehensive explanation of finding the partial derivative of the function
Finding the Partial Derivative of
Exploring the world of partial derivatives opens doors to advanced mathematical understanding. In this guide, we focus on finding the partial derivative of the function
| Indicate that we are taking the derivative with respect to |
|
| Apply the chain rule by multiplying the derivative of the inside function |
|
| Differentiate |
|
| Since we are taking the partial derivative with respect to |
|
| Write the final expression for the partial derivative of |
This step-by-step guide offers a detailed and clear explanation of finding the partial derivative of the function