The Integral of \( \frac{1}{1 + x^2} \) from Negative Infinity to Positive Infinity

When confronted with the idea of calculating a sum across an infinite range, many people are understandably perplexed. In calculus, we tackle this complex issue through a special kind of calculation known as an ‘improper integral.’ A quintessential example is \( \int_{-\infty}^{\infty} \frac{1}{1 + x^2} \, dx \).

Why Should You Care?

This improper integral is far from being merely an abstract concept; it frequently appears in various scientific disciplines such as physics, engineering, and statistics. Understanding its behavior—whether it converges or diverges—can have real-world applications.

Step 1: Addressing the Issue of Infinity

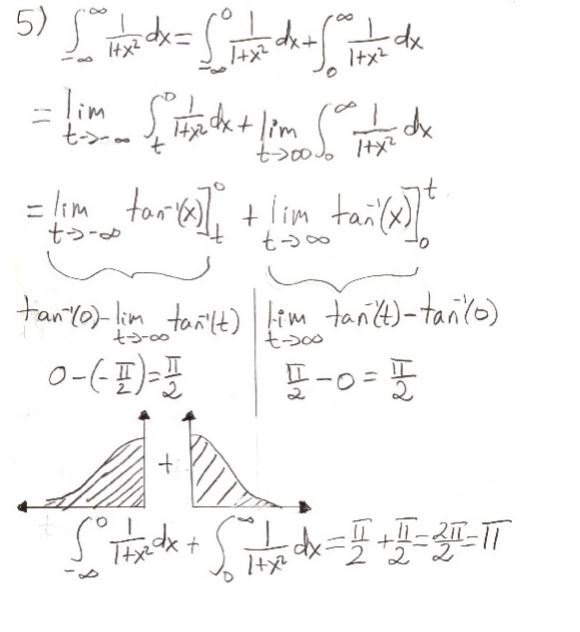
Since infinity is not a number but a concept, we cannot directly plug it into our integral. Thus, we introduce a new variable, \( b \), which serves as a temporary upper and lower bound for our integral. We then let \( b \) approach infinity and study the behavior of the integral:

\( \lim_{b \to \infty} \int_{-b}^{b} \frac{1}{1 + x^2} \, dx \)

Step 2: Finding the Antiderivative

The next vital step in solving any definite integral is to find its antiderivative. In this case, the antiderivative of \( \frac{1}{1 + x^2} \) is \( \arctan(x) \). How do we know this? By performing the reverse operation, differentiation, on \( \arctan(x) \), we confirm that the derivative is \( \frac{1}{1 + x^2} \).

\( \int \frac{1}{1 + x^2} \, dx = \arctan(x) + C \), where \( C \) is the constant of integration.

Step 3: Evaluating at the Boundaries

We now substitute the variable bounds \( -b \) and \( b \) into \( \arctan(x) \) and evaluate:

\( \arctan(b) – \arctan(-b) \)

Remember that \( \arctan(-x) = -\arctan(x) \). Therefore, we simplify this to \( 2\arctan(b) \).

Step 4: Examining the Limit

Now we apply the limit as \( b \) approaches infinity to \( 2\arctan(b) \).

\( \lim_{b \to \infty} 2\arctan(b) \)

Step 5: Convergence Check

The ultimate question is: does this limit converge to a finite value? To answer this, we look at the behavior of \( \arctan(b) \) as \( b \) approaches infinity. \( \arctan(b) \) approaches \( \frac{\pi}{2} \) as \( b \) goes to infinity. Consequently, \( 2\arctan(b) \) approaches \( \pi \).

Thus, \( \lim_{b \to \infty} 2\arctan(b) = \pi \), indicating that our original integral \( \int_{-\infty}^{\infty} \frac{1}{1 + x^2} \, dx \) converges to \( \pi \).

Final Thoughts

This integral demonstrates calculus’ unparalleled capability to take abstract, infinitely large concepts and derive concrete, finite solutions. And not just any solution—the integral converges to \( \pi \), one of the most significant numbers in mathematics.

What’s Next?

If you find this integral fascinating, there’s an entire universe of complex and intriguing integrals awaiting you in the world of calculus. The applications are virtually limitless, so keep exploring!