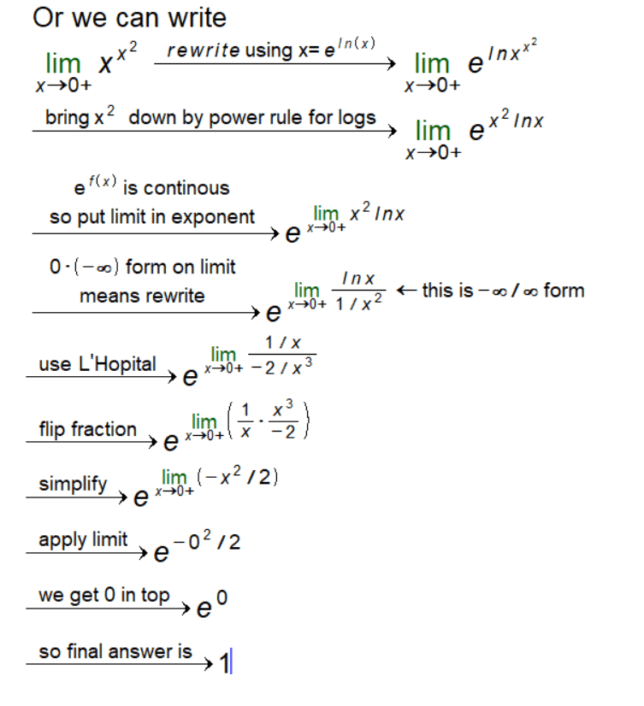
Finding the Limit of \( x^{x^2} \) as \( x \) Approaches 0

Let’s methodically work through the steps to find the limit of \( x^{x^2} \) as \( x \) approaches 0 from the positive side.

Step 1: The Objective

We want to find \( \lim_{{x \to 0^+}} x^{x^2} \).

Step 2: Change of Base

First, rewrite \( x \) as \( e^{\ln(x)} \): \[ x^{x^2} = (e^{\ln(x)})^{x^2} = e^{x^2 \ln(x)} \]

Step 3: The Exponent’s Challenge

The exponent \( x^2 \ln(x) \) is problematic. As \( x \) approaches 0, \( x^2 \) goes to 0 and \( \ln(x) \) goes to \(-\infty\), resulting in \(0 \times (-\infty)\).

Step 4: Preparing for L’Hôpital’s Rule

Rewrite \( x^2 \ln(x) \) as \( \frac{\ln(x)}{1/x^2} \): \[ \lim_{{x \to 0^+}} \frac{\ln(x)}{1/x^2} = \lim_{{x \to 0^+}} \frac{-\infty}{\infty} \]

Step 5: L’Hôpital’s Rule to the Rescue

Use L’Hôpital’s Rule to take the derivative of the numerator and the derivative of the denominator: \[ \lim_{{x \to 0^+}} \frac{d(\ln(x))/dx}{d(1/x^2)/dx} = \lim_{{x \to 0^+}} \frac{1/x}{-2/x^3} = \lim_{{x \to 0^+}} \frac{-x^2}{2} = 0 \] This resolves the \(-\infty/\infty\) indeterminacy and shows that \( x^2 \ln(x) \) tends to 0.

Step 6: Conclusion

Since \( x^2 \ln(x) \) tends to 0, \( e^{x^2 \ln(x)} \) tends to \( e^0 = 1 \): \[ \lim_{{x \to 0^+}} x^{x^2} = 1 \]

With this comprehensive step-by-step guide, we can confidently state that the limit of \( x^{x^2} \) as \( x \) approaches 0 from the positive side is 1.