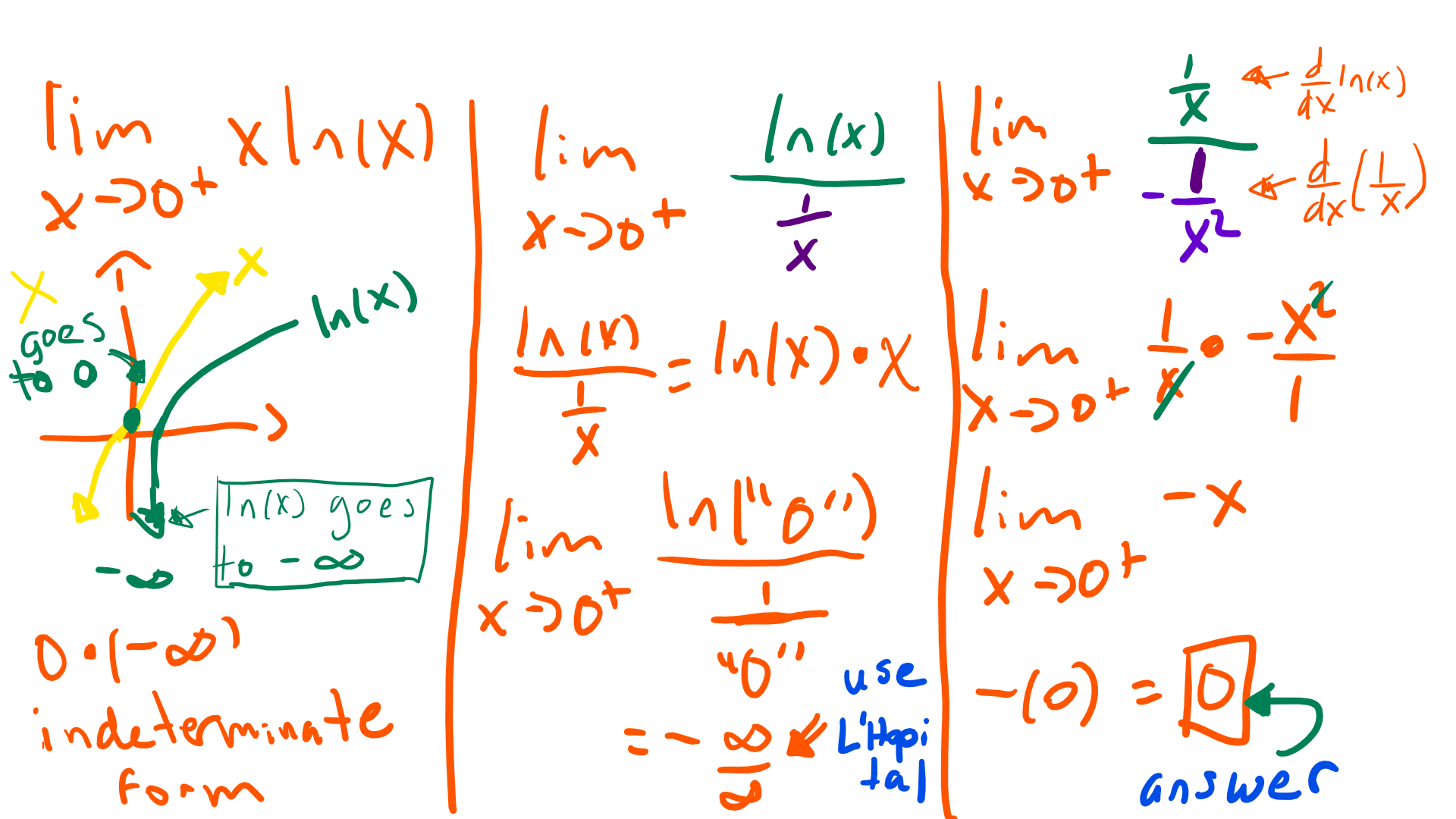
Original Expression: x ln x // Given expression

Limit: lim(x → 0⁺) x ln x // Limit as x approaches 0 from the right

Step 1: Recognize the indeterminate form: 0 ⋅ (-∞) // Identifying the indeterminate form

Step 2: Rewrite the expression as a fraction:

a. Rewrite as ln x / (1/x) // Rewriting the expression

Step 3: Apply L’Hôpital’s Rule:

a. Differentiate the numerator: d/dx ln x = 1/x // Differentiating the numerator

b. Differentiate the denominator: d/dx (1/x) = -1/x² // Differentiating the denominator

c. Rewrite the limit with derivatives: lim(x → 0⁺) (1/x) / (-1/x²) // Rewriting the limit

d. Simplify the expression: x² / -x // Simplifying the expression

e. Further simplify: -x² / x = -x // Further simplifying

f. Evaluate the limit: lim(x → 0⁺) -x = 0 // Evaluating the limit

Final Limit: lim(x → 0⁺) x ln x = 0 // Final result

---

---

---