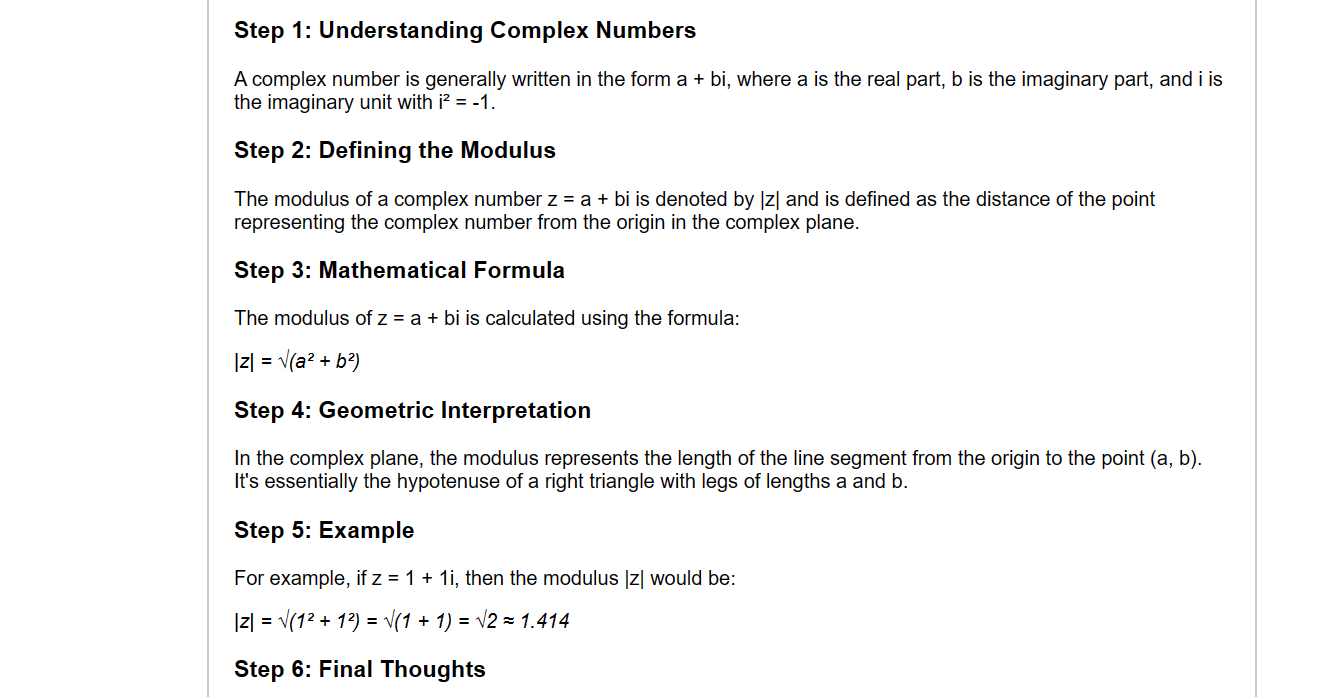
Step 1: Understanding Complex Numbers
A complex number is generally written in the form a + bi, where a is the real part, b is the imaginary part, and i is the imaginary unit with i² = -1.
Step 2: Defining the Modulus
The modulus of a complex number z = a + bi is denoted by |z| and is defined as the distance of the point representing the complex number from the origin in the complex plane.
Step 3: Mathematical Formula
The modulus of z = a + bi is calculated using the formula:
|z| = √(a² + b²)
Step 4: Geometric Interpretation
In the complex plane, the modulus represents the length of the line segment from the origin to the point (a, b). It’s essentially the hypotenuse of a right triangle with legs of lengths a and b.
Step 5: Example
For example, if z = 1 + 1i, then the modulus |z| would be:
|z| = √(1² + 1²) = √(1 + 1) = √2 ≈ 1.414
Step 6: Final Thoughts
The modulus is a crucial concept in complex number theory and has applications in various fields like engineering, physics, and signal processing.

Step 1: Understanding Complex Numbers
A complex number is generally written in the form \( a + bi \), where \( a \) is the real part, \( b \) is the imaginary part, and \( i \) is the imaginary unit with \( i^2 = -1 \).
Step 2: Defining the Modulus
The modulus of a complex number \( z = a + bi \) is denoted by \( |z| \) and is defined as the distance of the point representing the complex number from the origin in the complex plane.
Step 3: Mathematical Formula
The modulus of \( z = a + bi \) is calculated using the formula:
\[ |z| = \sqrt{a^2 + b^2} \]
Step 4: Geometric Interpretation
In the complex plane, the modulus represents the length of the line segment from the origin to the point \( (a, b) \). It’s essentially the hypotenuse of a right triangle with legs of lengths \( a \) and \( b \).
Step 5: Example
For example, if \( z = 3 + 4i \), then the modulus \( |z| \) would be:
\[ |z| = \sqrt{3^2 + 4^2} = \sqrt{9 + 16} = \sqrt{25} = 5 \]
Step 6: Final Thoughts
The modulus is a crucial concept in complex number theory and has applications in various fields like engineering, physics, and signal processing.
Step 1: Understanding Complex Numbers
A complex number is generally written in the form \( a + bi \), where \( a \) is the real part, \( b \) is the imaginary part, and \( i \) is the imaginary unit with \( i^2 = -1 \).
Step 2: Defining the Modulus
The modulus of a complex number \( z = a + bi \) is denoted by \( |z| \) and is defined as the distance of the point representing the complex number from the origin in the complex plane.
Step 3: Mathematical Formula
The modulus of \( z = a + bi \) is calculated using the formula:
\[ |z| = \sqrt{a^2 + b^2} \]
Step 4: Geometric Interpretation
In the complex plane, the modulus represents the length of the line segment from the origin to the point \( (a, b) \). It’s essentially the hypotenuse of a right triangle with legs of lengths \( a \) and \( b \).
Step 5: Example
For example, if \( z = \frac{1}{2} + \frac{1}{3}i \), then the modulus \( |z| \) would be:
\[ |z| = \sqrt{\left(\frac{1}{2}\right)^2 + \left(\frac{1}{3}\right)^2} = \sqrt{\frac{1}{4} + \frac{1}{9}} = \sqrt{\frac{9 + 4}{36}} = \sqrt{\frac{13}{36}} = \frac{\sqrt{13}}{6} \]
Step 6: Final Thoughts
The modulus is a crucial concept in complex number theory and has applications in various fields like engineering, physics, and signal processing.