Solving the Equation cos(x) = sin(x)
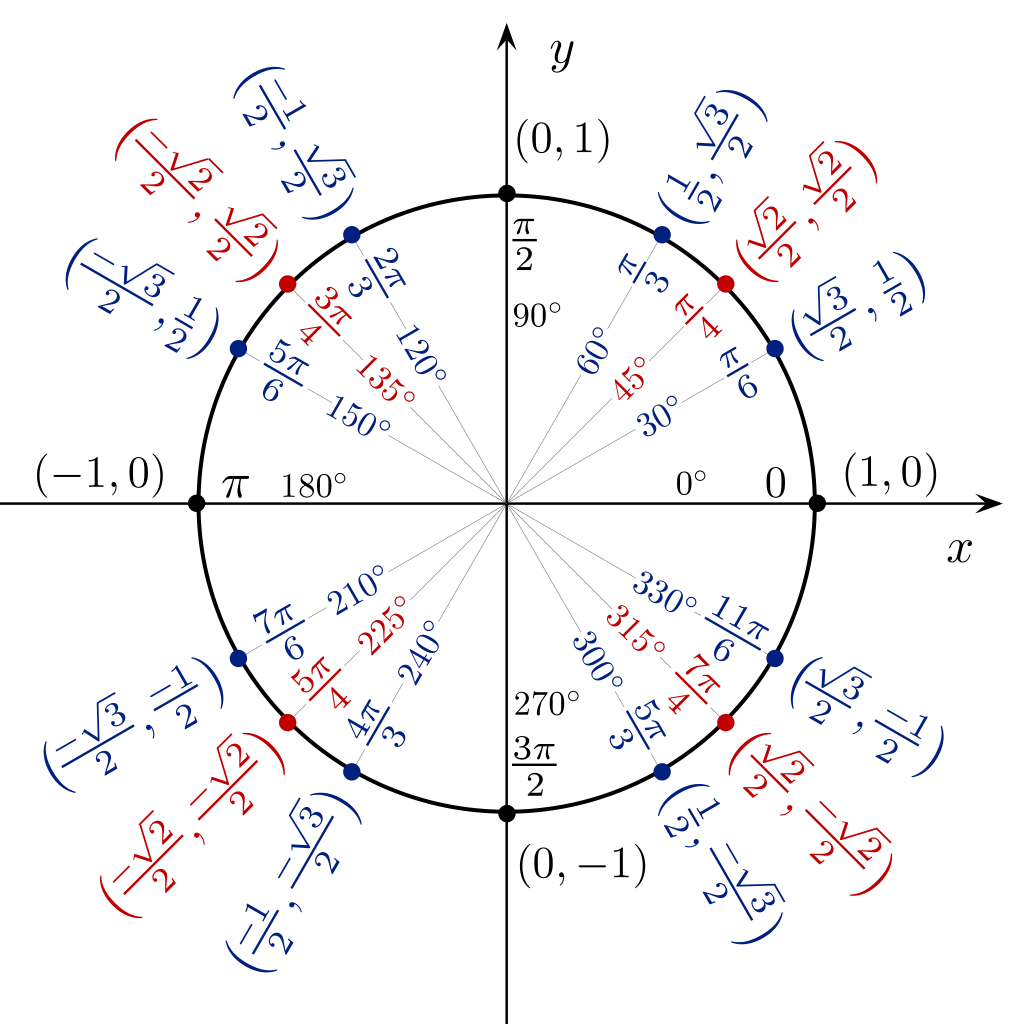
To solve the equation \(\cos(x) = \sin(x)\), we can think about the unit circle. On the unit circle, the x-coordinate represents the cosine of the angle and the y-coordinate represents the sine of the angle.
The equation \(\cos(x) = \sin(x)\) represents the points on the unit circle where the x-coordinate (cosine) is equal to the y-coordinate (sine). This happens at two points on the unit circle: one in the first quadrant and one in the third quadrant.
In the first quadrant, the angle is \(x = \frac{\pi}{4}\), because at this angle, both the x-coordinate and the y-coordinate are \(\frac{\sqrt{2}}{2}\), which means the cosine and sine of the angle are equal.
In the third quadrant, the angle is \(x = \frac{5\pi}{4}\), because at this angle, both the x-coordinate and the y-coordinate are \(-\frac{\sqrt{2}}{2}\), which again means the cosine and sine of the angle are equal.
So, the solutions to the equation \(\cos(x) = \sin(x)\) in the interval \([0, 2\pi)\) are \(x = \frac{\pi}{4}\) and \(x = \frac{5\pi}{4}\).
Here is a diagram of the unit circle with the angles marked:
And here is a plot of the functions \(\cos(x)\) and \(\sin(x)\) in the interval \([0, 2\pi)\), with the solutions marked in red:
Solving the Equation cos(x) = -sin(x)
To solve the equation \(\cos(x) = -\sin(x)\), we can think about the unit circle. On the unit circle, the x-coordinate represents the cosine of the angle and the y-coordinate represents the sine of the angle.
The equation \(\cos(x) = -\sin(x)\) represents the points on the unit circle where the x-coordinate (cosine) is equal to the negative of the y-coordinate (sine). This happens at two points on the unit circle: one in the second quadrant and one in the fourth quadrant.
In the second quadrant, the angle is \(x = \frac{3\pi}{4}\), because at this angle, the x-coordinate is \(-\frac{\sqrt{2}}{2}\) and the y-coordinate is \(\frac{\sqrt{2}}{2}\), which means the cosine of the angle is equal to the negative of the sine of the angle.
In the fourth quadrant, the angle is \(x = \frac{7\pi}{4}\), because at this angle, the x-coordinate is \(\frac{\sqrt{2}}{2}\) and the y-coordinate is \(-\frac{\sqrt{2}}{2}\), which again means the cosine of the angle is equal to the negative of the sine of the angle.
So, the solutions to the equation \(\cos(x) = -\sin(x)\) in the interval \([0, 2\pi)\) are \(x = \frac{3\pi}{4}\) and \(x = \frac{7\pi}{4}\).
Here is a diagram of the unit circle with the angles marked:

And here is a plot of the functions \(\cos(x)\) and \(-\sin(x)\) in the interval \([0, 2\pi)\), with the solutions marked in red:
Solving the Equation cot(x) = tan(x)
To solve the equation \( \cot(x) = \tan(x) \), we can think about the unit circle. On the unit circle, the x-coordinate represents the cosine of the angle and the y-coordinate represents the sine of the angle.
The equation \( \cot(x) = \tan(x) \) represents the points on the unit circle where the ratio of the x-coordinate to the y-coordinate (cotangent) is equal to the ratio of the y-coordinate to the x-coordinate (tangent). This happens at two points on the unit circle: one in the first quadrant and one in the third quadrant.
In the first quadrant, the angle is \( x = \frac{\pi}{4} \), because at this angle, the x-coordinate is \(\frac{\sqrt{2}}{2}\) and the y-coordinate is \(\frac{\sqrt{2}}{2}\), which means the cotangent of the angle is equal to the tangent of the angle.
In the third quadrant, the angle is \( x = -\frac{\pi}{4} \), because at this angle, the x-coordinate is \(-\frac{\sqrt{2}}{2}\) and the y-coordinate is \(-\frac{\sqrt{2}}{2}\), which again means the cotangent of the angle is equal to the tangent of the angle.
So, the solutions to the equation \( \cot(x) = \tan(x) \) in the interval \( -\frac{\pi}{2} < x < \frac{\pi}{2} \) are \( x = -\frac{\pi}{4} \) and \( x = \frac{\pi}{4} \).
Here is the graph of the functions \( \cot(x) \) and \( \tan(x) \) over the interval \( -\frac{\pi}{2} < x < \frac{\pi}{2} \):
