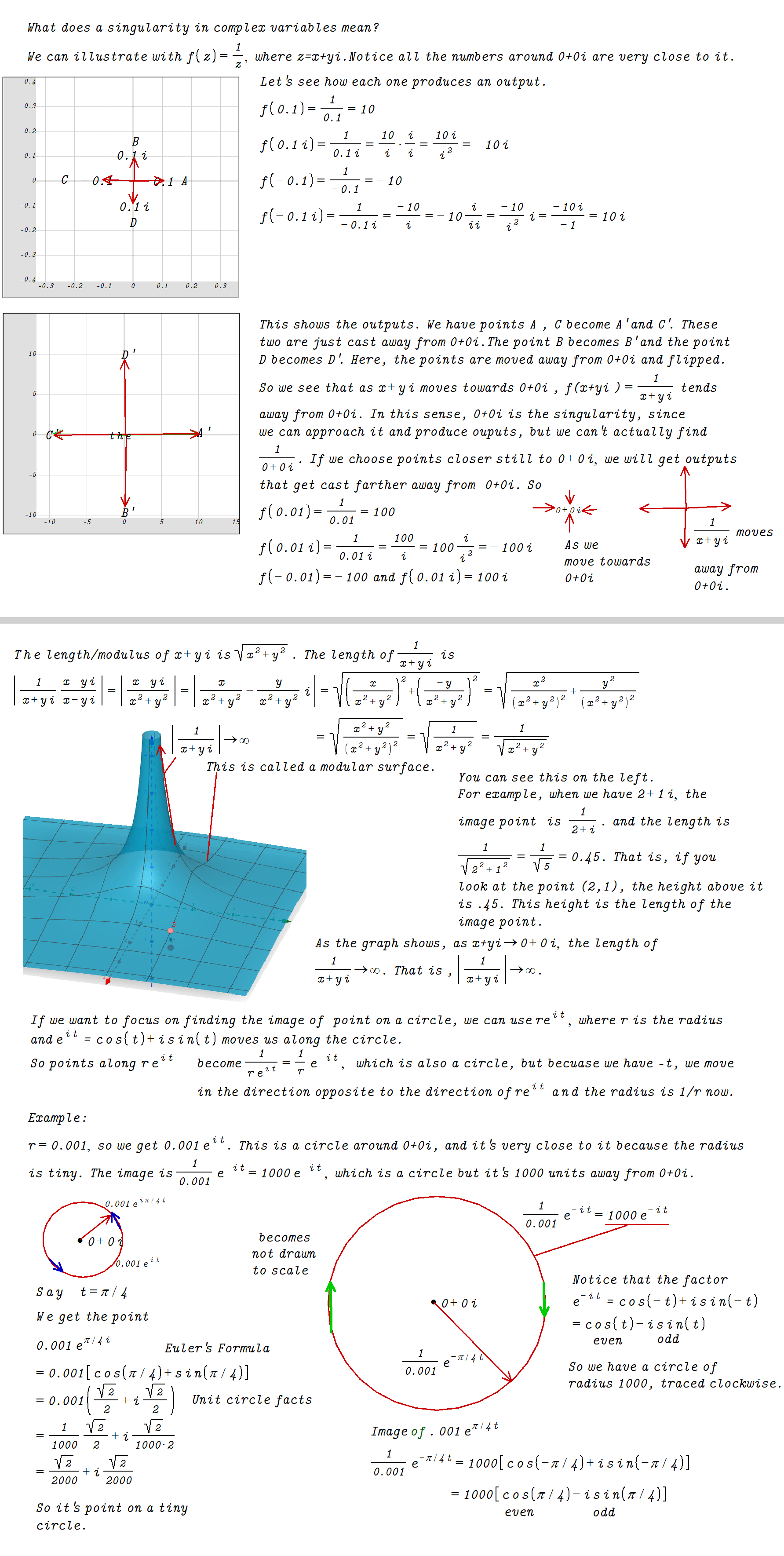
Explore the fascinating world of complex analysis with our interactive 3D plot of a simple pole. This visualization represents the function 1/z near z = 0, demonstrating the characteristic behavior of a pole as the modulus of the function’s value goes to infinity when approaching the origin. This behavior is consistent regardless of the path taken to the origin, whether it’s along the real axis, the imaginary axis, or any other path. Our detailed table of modulus computations further illustrates this concept, providing a clear and intuitive understanding of poles in complex analysis. Dive into the complex plane with us and discover the beauty of mathematical functions and their behaviors.
This is a simple pole. The function behaves like \(1/z\) near \(z = 0\). The height of the surface represents the modulus of the function’s value. The modulus goes to infinity as we approach the origin, which is a characteristic of a simple pole. This is true no matter how we approach the origin in the complex plane, whether it’s along the real axis, the imaginary axis, or any other path.
In the complex plane, a complex number \(z\) can be represented as \(x + iy\), where \(x\) is the real part and \(y\) is the imaginary part. The modulus (or absolute value) of \(z\) is given by \(\sqrt{x^2 + y^2}\).
The function \(f(z) = 1/z\) is a function with a simple pole at \(z = 0\). The modulus of this function is \(|f(z)| = 1/|z| = 1/\sqrt{x^2 + y^2}\). This means that as \(z\) approaches 0 (either along the real axis, the imaginary axis, or any other path), the modulus of \(f(z)\) goes to infinity. This is the characteristic of a pole.
If we approach 0 along the real axis (i.e., \(y = 0\)), then \(z = x\), and the modulus of \(f(z)\) becomes \(1/|x|\), which goes to infinity as \(x\) approaches 0. This is represented by the sharp peak in the 3D plot at the origin.
Similarly, if we approach 0 along the imaginary axis (i.e., \(x = 0\)), then \(z = iy\), and the modulus of \(f(z)\) becomes \(1/|y|\), which also goes to infinity as \(y\) approaches 0.
Modulus computations for \(1/z\) as \(z\) approaches 0 along the real axis:
| x | |1/x| |
|---|---|
| -0.1 | 10.0 |
| -0.08 | 12.5 |
| -0.06 | 16.67 |
| -0.04 | 25.0 |
| -0.02 | 50.0 |
| 0.0 | Infinity |
| 0.02 | 50.0 |
| 0.04 | 25.0 |
| 0.06 | 16.67 |
| 0.08 | 12.5 |
| 0.1 | 10.0 |