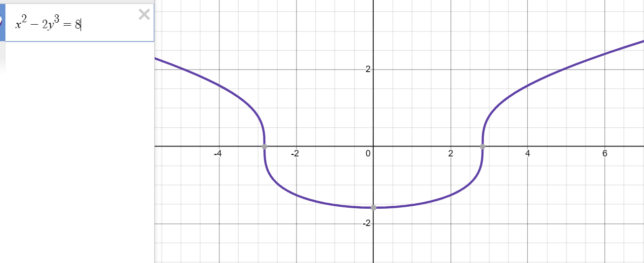
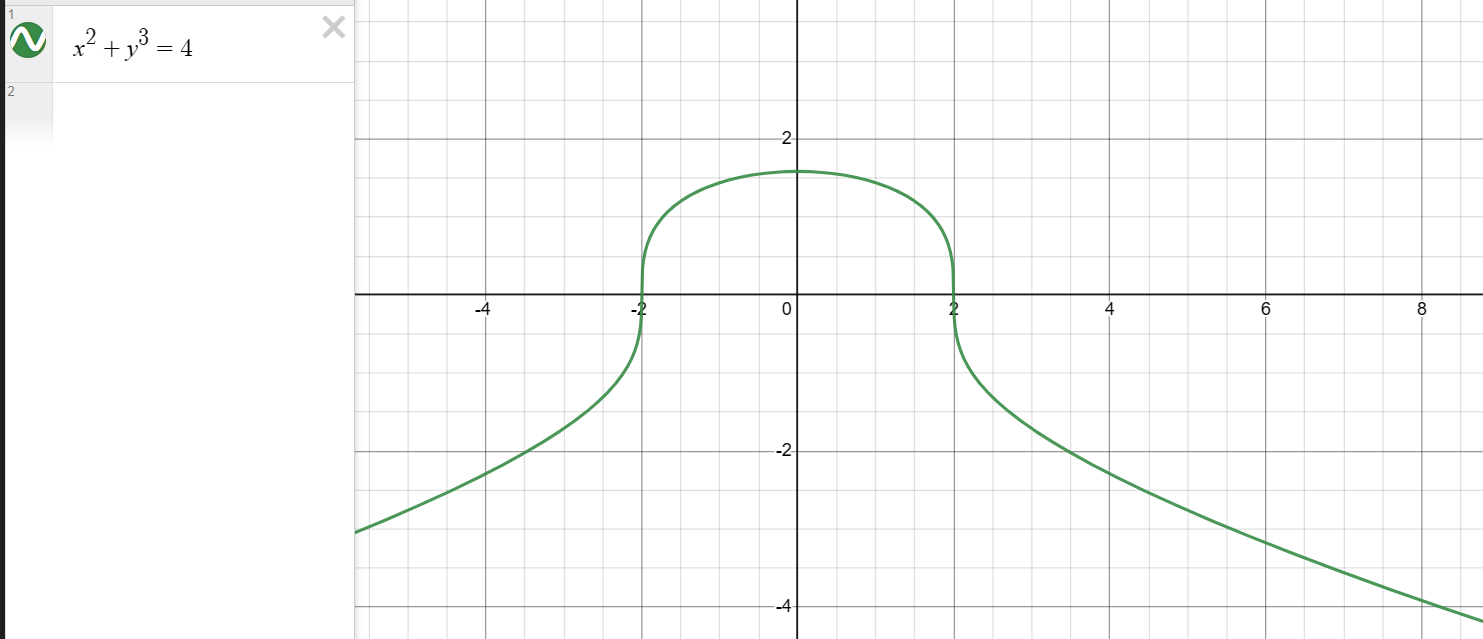
Implicit Differentiation of the Equation x² + y³ = 4
In calculus, implicit differentiation is a technique used to differentiate equations where one variable is not explicitly defined in terms of the other. In this case, we have the equation x² + y³ = 4, and we aim to find dy/dx, the derivative of y with respect to x.
Differentiating implicitly with respect to x, we get: 2x + 3y²(dy/dx) = 0
Now, solving for dy/dx, we can rearrange the terms to get: 3y²(dy/dx) = -2x
Finally, we find that dy/dx = -2x / (3y²)
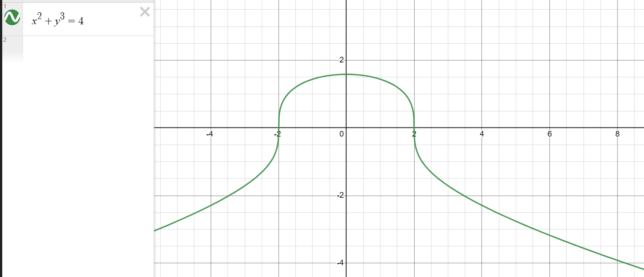
Implicit Differentiation of the Equation x² – 2y³ = 8
In this scenario, we are tasked with finding the derivative dy/dx for the given equation x² – 2y³ = 8 using implicit differentiation.
Step 1: Differentiate both sides of the equation with respect to x, applying the chain rule when necessary. This yields: 2x – 6y²(dy/dx) = 0.
Step 2: Move the term involving x to the right-hand side of the equation, resulting in: -6y²(dy/dx) = -2x.
Step 3: Now, to isolate dy/dx, divide both sides of the equation by -6y², which gives: dy/dx = -2x / (-6y²) = x / (3y²).