The Integration of the Exponential Function e^(-x)

Learn the integration of the exponential function e⁻ˣ. This guide provides the integral ∫e⁻ˣ dx = -e⁻ˣ + C, essential for calculus students.

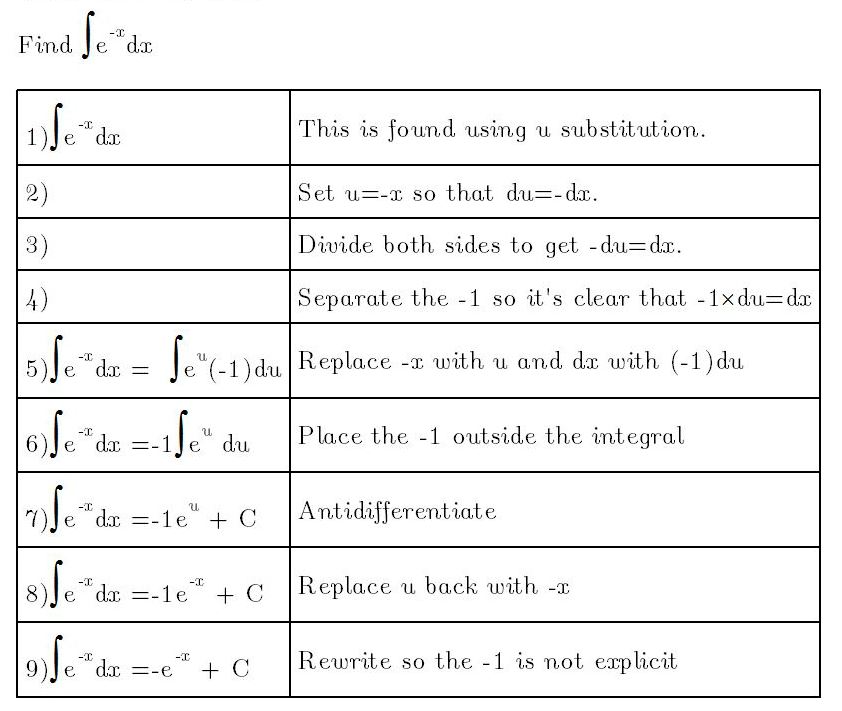
Integration of the Exponential Function $ e^{-x} $: Step-by-Step Guide

Step 1: Identify the Integral

Math: $\int e^{-x} \,dx$

Explanation: The integration of the exponential function $ e^{-x} $ begins by identifying the function we want to integrate. This is the starting point for understanding how to perform the integration of the exponential function $ e^{-x} $.

Step 2: Apply a Substitution

Math: $u = -x, du = -dx$

Explanation: In the integration of the exponential function $ e^{-x} $, a substitution is made to simplify the expression. This substitution is a key step in the integration of the exponential function $ e^{-x} $.

Step 3: Rewrite the Integral in Terms of $ u $

Math: $\int e^{u} \,du$

Explanation: The integral is rewritten in terms of $ u $, a crucial step in the integration of the exponential function $ e^{-x} $.

Step 4: Divide by -1

Math: $-\int e^{u} \,du$

Explanation: Since $ du = -dx $, we divide both sides of the integral by -1. This division is a vital algebraic step in the integration of the exponential function $ e^{-x} $.

Step 5: Integrate the Exponential Function

Math: $-e^{u} + C$

Explanation: The exponential function $ e^{u} $ is integrated with respect to $ u $, resulting in $-e^{u} + C$. This integration is a core part of the integration of the exponential function $ e^{-x} $.

Step 6: Substitute Back for $ x $

Math: $-e^{-x} + C$

Explanation: The final step in the integration of the exponential function $ e^{-x} $ is to substitute back for $ x $, completing the process.

Final Result

Math: $\int e^{-x} \,dx = -e^{-x} + C$

Explanation: The integration of the exponential function $ e^{-x} $ results in $-e^{-x} + C$, where $ C $ is the constant of integration. This detailed guide illustrates every algebraic step in the integration of the exponential function $ e^{-x} $, making it a comprehensive resource for anyone looking to understand this mathematical concept.

Conclusion

The integration of the exponential function $ e^{-x} $ is a fundamental concept in calculus. This step-by-step guide provides a clear and detailed explanation, ensuring a deep understanding of the integration of the exponential function $ e^{-x} $. Whether you are a student, educator, or math enthusiast, this guide to the integration of the exponential function $ e^{-x} $ offers valuable insights and clarity.

antiderivative of e^(-x)

Steps to using this site for homework help:
1. Provide a detailed description of what you need in the form below.
2. Read the terms of service before clicking Submit.
3. Click Submit.
4. Make a payment of $4 for your problem on the next page using PayPal.
5. If we can solve your problem, we will send the solutions via email.
6. If we cannot solve your problem, you’ll receive a refund promptly.

students-1617762

Click here to view the terms of service. You agree to the terms when you click Submit.

Terms of Service:
Read these carefully before agreeing to them.
1. You accept this service as-is.
2. You understand that we do not guarantee your problem can be solved.
3. If your problem cannot be solved, we’ll communicate this to you.
4. You understand that there is no guarantee that the solution provided is 100% correct.
5. If a solution is not correct, you’re entitled to a refund with 7 days.
6. If you want another solution, you must pay again after the refund.
7. If a solution cannot be provided, you’re entitled to a refund within 7 days.
8. You promise not to hold the owners of www.calculuscoaches.com, or masterskills.net, responsible for incorrect solutions, or solutions that cannot be provided.
9. You promise to indemnify www.calculuscoaches.com, and masterskills.net, against any other undesirable effect or claim that might arise from the use of this site. In other words, you have no legal claim against us if the solutions are not correct, or cannot be provided, or something happens with your institution or instructor.
10. You promise not to upload files that contain any racist, homophobic or pornographic content.
11. You promise not to upload files that could in any way harm the dignity of the problem solver.
12. You promise not to upload files that could damage the operation of this site.
13. You understand that payment for services rendered must be made in advance.
14. You understand that payment is made via PayPal only.
15. We collect your first name, last name and email address for communication purposes only.
16. We do not share any information collected with any third parties.
17. You may link to this site, but only if your link will not damage our reputation.
18. You are encouraged to read and understand the solution provided, and not merely claim it as your own. This is why our solutions are annotated carefully.
19. You understand that we will do our best to provide you with a carefully worded, correct solution within as short a period of time as possible.
20. You agree to receive the solution in your email.

Integration of the Exponential Function \( e^{-x} \): Step-by-Step Guide