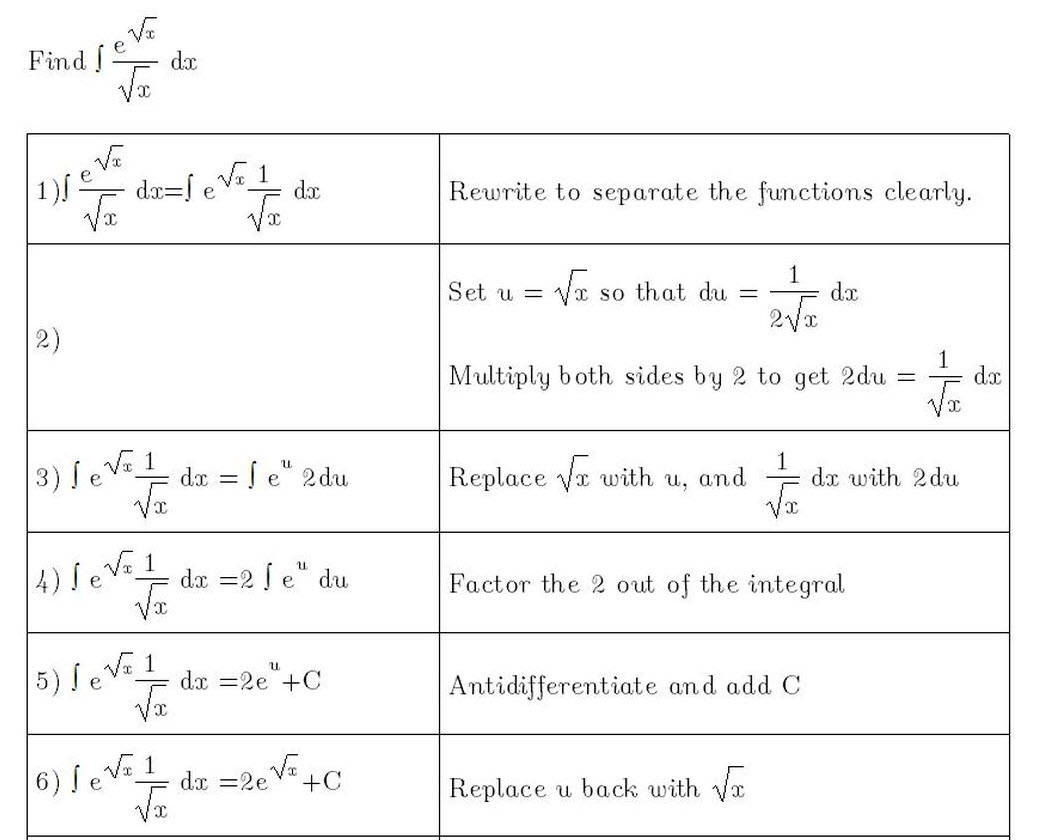
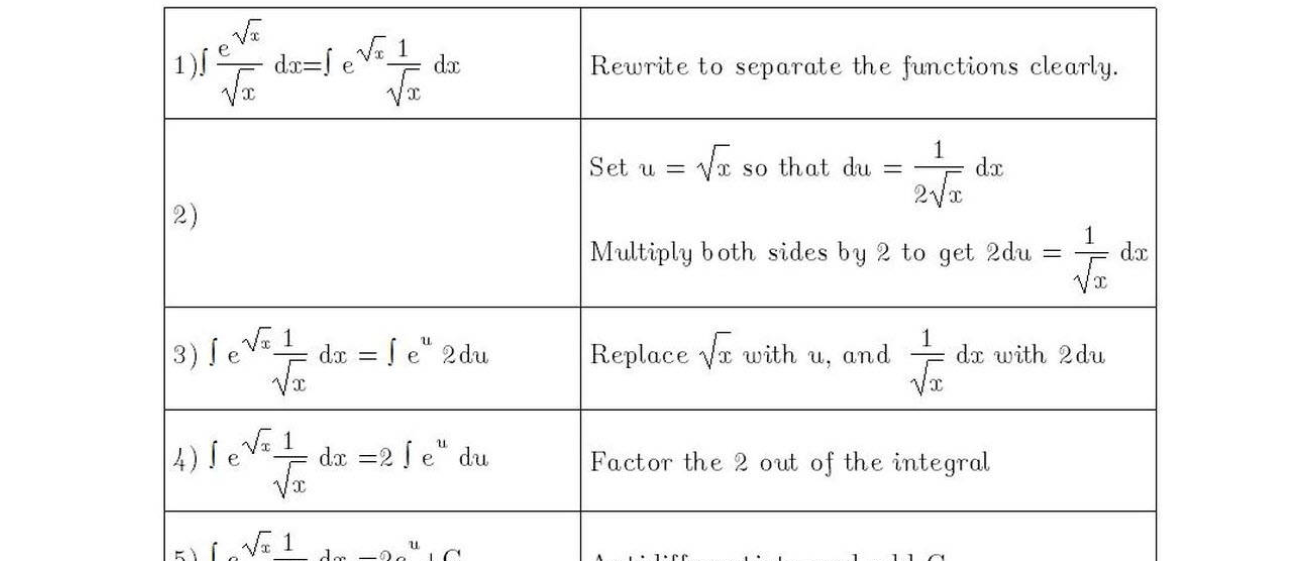
We are exploring the antiderivative of the mathematical expression e√x / √x. By employing a variable substitution (u = √x), we can simplify the expression and find the derivative du/dx. Through a series of algebraic manipulations, we isolate dx and replace it in our original integral, leading to a simplified form that can be easily integrated. The final result is an expression for the antiderivative in terms of the original variable x: 2e√x + C. This guide breaks down every step of the process, providing detailed explanations suitable for those new to calculus.
  1. Choose a substitution \( u = \sqrt{x} \), so we can replace \( \sqrt{x} \) with \( u \).
  2. Differentiate both sides to find \( \frac{du}{dx} = \frac{1}{2\sqrt{x}} \), which tells us how \( u \) changes with respect to \( x \).
  3. Multiply both sides of the above equation by \( dx \) to isolate \( du \): \( du = \frac{1}{2\sqrt{x}} \cdot dx \).
  4. Now, replace \( \sqrt{x} \) with \( u \) in the previous step: \( du = \frac{1}{2u} \cdot dx \).
  5. Multiply both sides by \( 2u \) to solve for \( dx \): \( 2u \cdot du = dx \).
  6. Substitute \( dx \), \( \sqrt{x} \), and \( \frac{e^{\sqrt{x}}}{\sqrt{x}} \) in the original integral with the expressions found earlier: \( \int \frac{e^u}{u} \cdot 2u \, du \).
  7. Simplify by canceling \( u \) in the numerator and denominator: \( 2\int e^u \, du \).
  8. Take the integral of \( e^u \) with respect to \( u \): \( 2e^u + C \).
  9. Substitute back the original expression for \( u \): \( 2e^{\sqrt{x}} + C \).
  10. The final result is the antiderivative of \( \frac{e^{\sqrt{x}}}{\sqrt{x}} \): \( 2e^{\sqrt{x}} + C \).
  11. This function represents all the possible antiderivatives of the original function, differing only by a constant \( C \).

antiderivative of e to the square root of x over the square root of x