How to Find the Antiderivative of 1/x²: A Comprehensive Step-by-Step Guide

Introduction: Finding the antiderivative of a function is a fundamental concept in calculus. In this guide, we will explore the process of finding the antiderivative of 1/x², a common mathematical expression.

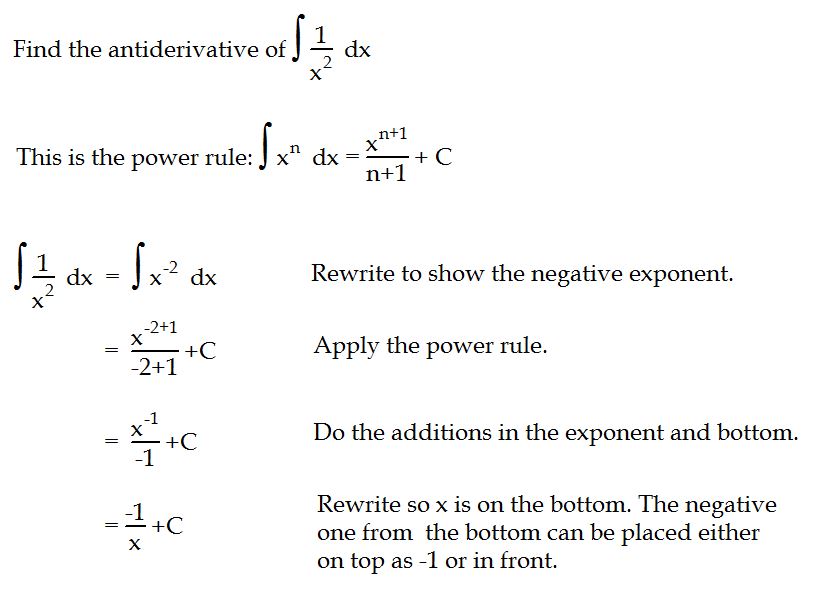
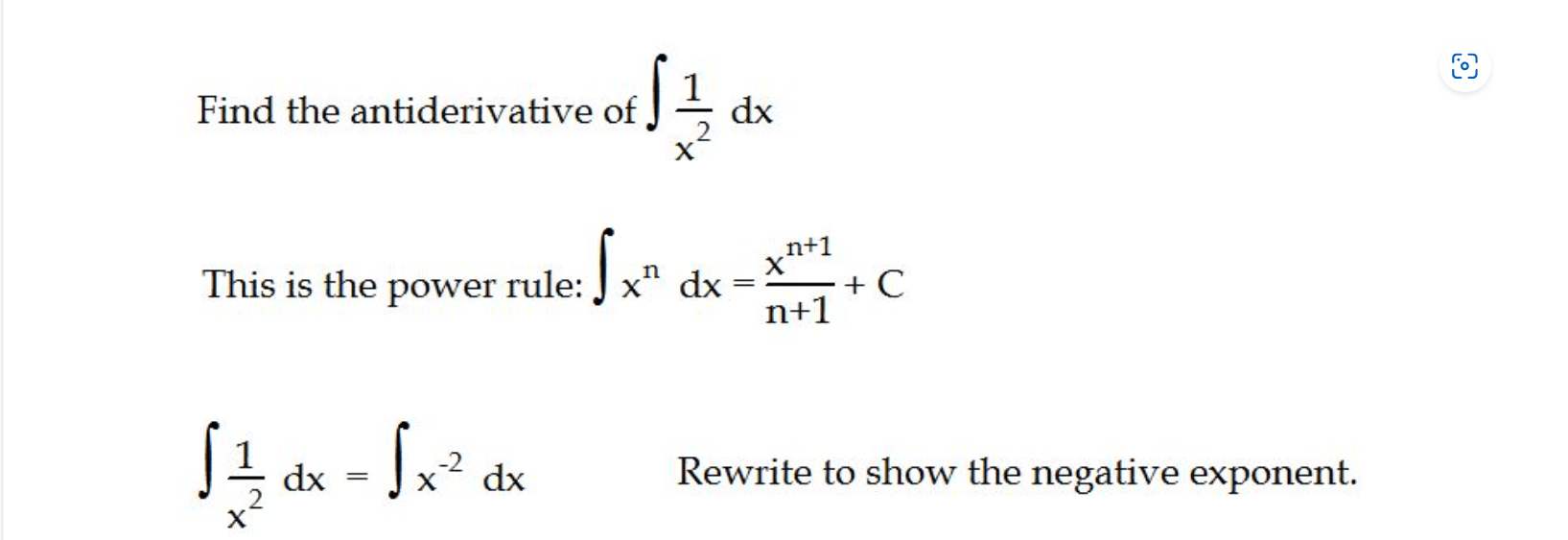
Step 1: Rewrite the Expression

We begin by rewriting the given expression in a more manageable form. The expression 1/x² can be expressed as x⁻², where the exponent -2 indicates the reciprocal of the square of x.

Step 2: Write the Integral

Next, we write down the integral we want to find. The integral of 1/x² with respect to x is represented as ∫ 1/x² dx, or equivalently, ∫ x⁻² dx.

Step 3: Apply the Power Rule for Integration

The power rule for integration is a fundamental tool in calculus. It states that the antiderivative of xⁿ is (xⁿ⁺¹)/(n+1) + C, where C is the constant of integration. Applying this rule to our expression, with n = -2, we find:

∫ x⁻² dx = (x⁻¹)/(-1) + C = -1/x + C

Conclusion: The antiderivative of 1/x² is -1/x + C. This result is essential in various applications, including physics, engineering, and economics. Understanding how to find the antiderivative of functions like 1/x² is a valuable skill in mathematics and related fields.