assignment 7, question 4
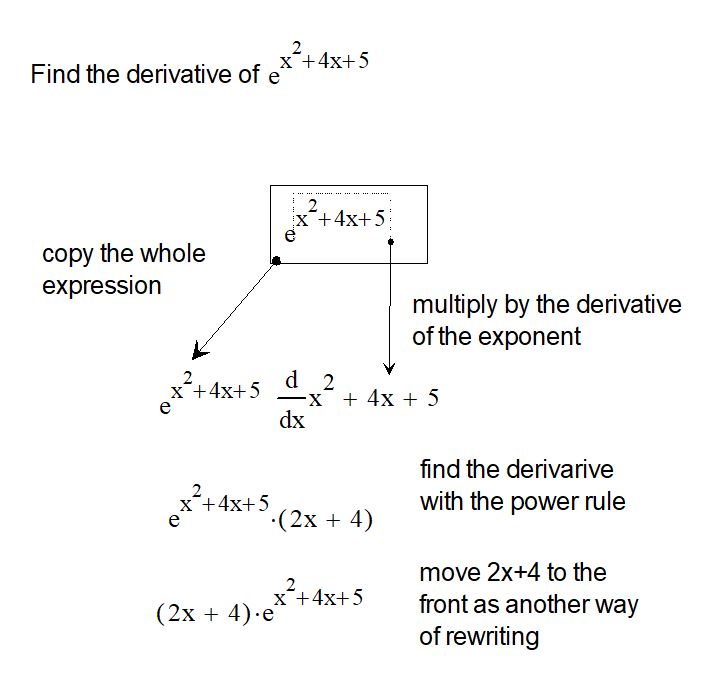
Derivative of \( e^{x^2 + 4x + 5} \) Explained
| Mathematical Steps | Explanation |
|---|---|
| Identify \( f(g(x)) \) and \( g(x) \) | \( f(g(x)) = e^{g(x)} \) and \( g(x) = x^2 + 4x + 5 \) |
| Find \( f'(g(x)) \) | \( f'(g(x)) = e^{g(x)} \) |
| Find \( g'(x) \) | \( g'(x) = 2x + 4 \) |
| Apply the Chain Rule | \( \frac{d}{dx}[e^{x^2 + 4x + 5}] = e^{x^2 + 4x + 5} \times (2x + 4) \) |
| Simplify | \( \frac{d}{dx}[e^{x^2 + 4x + 5}] = (2x + 4)e^{x^2 + 4x + 5} \) |
Final Result
The derivative of \( e^{x^2 + 4x + 5} \) is \( (2x + 4)e^{x^2 + 4x + 5} \).
Derivative of \( e^{x^2 + 4x + 5} \) Explained
| Mathematical Steps | Explanation |
|---|---|
| Identify \( f(g(x)) \) and \( g(x) \) | We start by identifying the inner function \( g(x) = x^2 + 4x + 5 \) and the outer function \( f(g(x)) = e^{g(x)} \). |
| Find \( f'(g(x)) \) | The derivative of \( e^{g(x)} \) with respect to \( g(x) \) is \( e^{g(x)} \). |
| Find \( g'(x) \) | The derivative of \( x^2 + 4x + 5 \) with respect to \( x \) is \( 2x + 4 \). |
| Apply the Chain Rule | Using the chain rule, we find that \( \frac{d}{dx}[e^{x^2 + 4x + 5}] = e^{x^2 + 4x + 5} \times (2x + 4) \). |
| Simplify | Finally, we simplify the expression to \( (2x + 4)e^{x^2 + 4x + 5} \). |
Final Result
The derivative of \( e^{x^2 + 4x + 5} \) is \( (2x + 4)e^{x^2 + 4x + 5} \).
