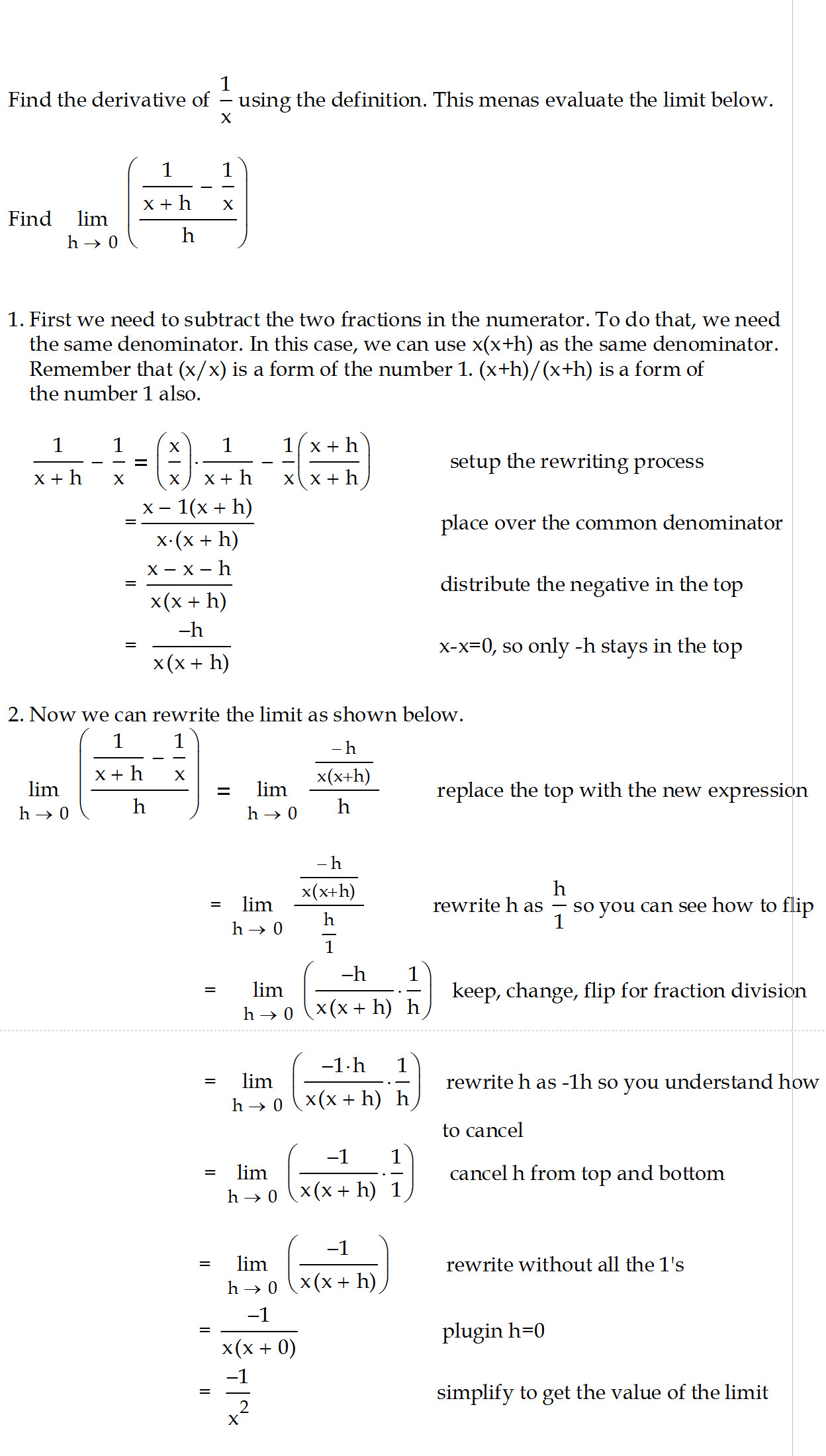
Embark on a Mathematical Exploration: Unraveling the Derivative of 1/x Using the Definition

Step 1: Start with the definition of the derivative: f'(x) = lim(h → 0) (f(x + h) – f(x))/h

Step 2: Substitute the function f(x) = 1/x into the definition.

Step 3: Combine the fractions in the numerator to get: -h/(h(x + h)x)

Step 4: Simplify the expression by canceling h: -1/((x + h)x)

Step 5: Take the limit as h approaches 0: -1/x²

Conclusion: The derivative of 1/x is -1/x². This result is derived using the fundamental definition of the derivative, showcasing the elegance and precision of calculus.