Uncover the intricacies of calculus with our comprehensive guide on finding the derivatives of the natural logarithm of sine, ln(sin(x)), and cosine, ln(cos(x)). We present the process in two distinct formats to cater to different learning styles. For those who prefer a detailed walkthrough, we provide a step-by-step annotation of each stage, from applying the chain rule to multiplying the derivatives. For those who prefer a concise approach, we also offer an efficient, single-line format for quick reference. This guide is perfect for students, educators, and anyone with a keen interest in calculus, offering valuable insights regardless of your preferred learning style.
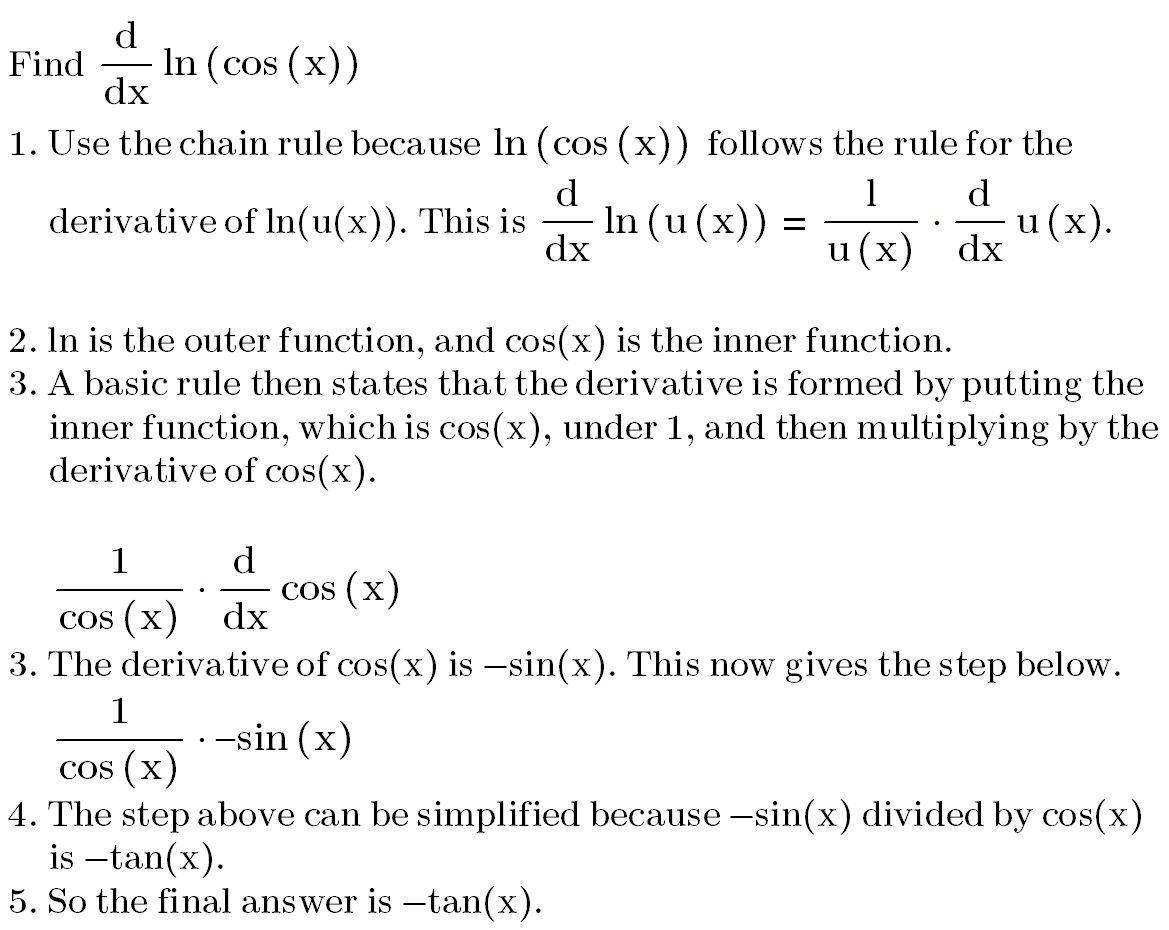
Step 1: Identify the function, which is \(ln(cos(x))\).
Step 2: Apply the chain rule. The chain rule states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function. Here, the outer function is \(ln(x)\) and the inner function is \(cos(x)\).
Step 3: Find the derivative of the outer function. The derivative of \(ln(x)\) is \(1/x\).
Step 4: Find the derivative of the inner function. The derivative of \(cos(x)\) is \(-sin(x)\).
Step 5: Multiply the derivatives. The derivative of \(ln(cos(x))\) is \(\frac{-sin(x)}{cos(x)}\) or \(-tan(x)\).
Step 1: Identify the function, which is \(ln(sin(x))\).
Step 2: Apply the chain rule. The chain rule states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function. Here, the outer function is \(ln(x)\) and the inner function is \(sin(x)\).
Step 3: Find the derivative of the outer function. The derivative of \(ln(x)\) is \(1/x\).
Step 4: Find the derivative of the inner function. The derivative of \(sin(x)\) is \(cos(x)\).
Step 5: Multiply the derivatives. The derivative of \(ln(sin(x))\) is \(\frac{cos(x)}{sin(x)}\) or \(cot(x)\).
Efficient Line: \(d/dx[ln(sin(x))]=1/sin(x) * d/dx[sin(x)]=1/sin(x) * cos(x)=cot(x)\)
| Step | Comment |
|---|---|
| Step 1 | Identify the function, which is \(ln(tan(x))\). |
| Step 2 | Apply the chain rule. The chain rule states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function. Here, the outer function is \(ln(x)\) and the inner function is \(tan(x)\). |
| Step 3 | Find the derivative of the outer function. The derivative of \(ln(x)\) is \(1/x\). |
| Step 4 | Find the derivative of the inner function. The derivative of \(tan(x)\) is \(sec^2(x)\). |
| Step 5 | Multiply the derivatives. The derivative of \(ln(tan(x))\) is \(\frac{sec^2(x)}{tan(x)}\). |
| Efficient Line | \(d/dx[ln(tan(x))]=1/tan(x) * d/dx[tan(x)]=1/tan(x) * sec^2(x)=sec^2(x)/tan(x)=csc(x)cos(x)\) |
| Step | Comment |
|---|---|
| Step 1 | Identify the function, which is \(ln(sec(x))\). |
| Step 2 | Apply the chain rule. The chain rule states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function. Here, the outer function is \(ln(x)\) and the inner function is \(sec(x)\). |
| Step 3 | Find the derivative of the outer function. The derivative of \(ln(x)\) is \(1/x\). |
| Step 4 | Find the derivative of the inner function. The derivative of \(sec(x)\) is \(sec(x)tan(x)\). |
| Step 5 | Multiply the derivatives. The derivative of \(ln(sec(x))\) is \(\frac{sec(x)tan(x)}{sec(x)}=tan(x)\). |
| Efficient Line | \(d/dx[ln(sec(x))]=1/sec(x) * d/dx[sec(x)]=1/sec(x) * sec(x)tan(x)=tan(x)\) |

