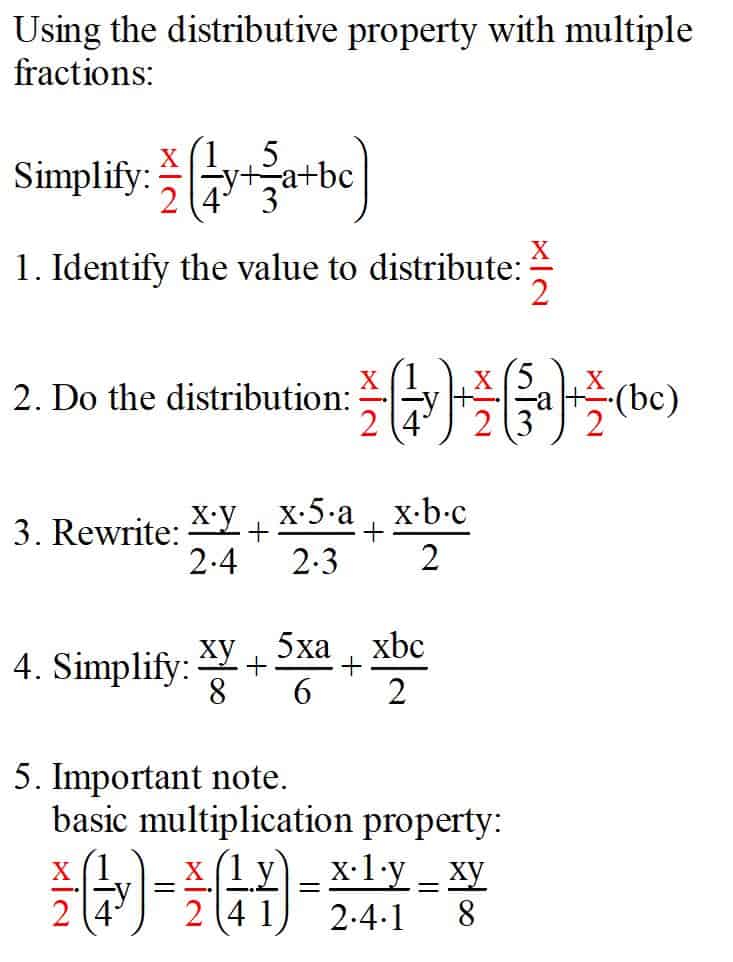
Comprehensive Solution for the Expression \( \frac{x}{2} \left( \frac{1}{4y} + \frac{5}{3a} + bc \right) \)

Step 1: Identify the Expression

We are given the expression \( \frac{x}{2} \left( \frac{1}{4y} + \frac{5}{3a} + bc \right) \).

Step 2: Distribute \( \frac{x}{2} \) to Each Term Inside the Parentheses

Our next step is to apply the distributive property to eliminate the parentheses. We will multiply \( \frac{x}{2} \) by each term inside \( \frac{1}{4y} + \frac{5}{3a} + bc \).

Expression: \( \frac{x}{2} \times \frac{1}{4y} + \frac{x}{2} \times \frac{5}{3a} + \frac{x}{2} \times bc \)

Step 3: Simplify Each Term

Now, we will simplify each term by multiplying the fractions.

First Term: \( \frac{x}{2} \times \frac{1}{4y} = \frac{x}{8y} \)

Second Term: \( \frac{x}{2} \times \frac{5}{3a} = \frac{5x}{6a} \)

Third Term: \( \frac{x}{2} \times bc = \frac{bcx}{2} \)

Step 4: Combine the Simplified Terms

Finally, we combine all the simplified terms to get the final expression.

Combined Expression: \( \frac{x}{8y} + \frac{5x}{6a} + \frac{bcx}{2} \)

Conclusion: The simplified expression for \( \frac{x}{2} \left( \frac{1}{4y} + \frac{5}{3a} + bc \right) \) is \( \frac{x}{8y} + \frac{5x}{6a} + \frac{bcx}{2} \).