Welcome to our Mathematics Examples Page! Here, you’ll find a curated collection of step-by-step mathematical solutions presented in plain text with real math symbols. From solving equations to applying the distributive property, each example is carefully crafted for clarity and ease of understanding. Whether you’re a student, teacher, or math enthusiast, these concise and informative examples will help you grasp mathematical concepts with confidence. Enjoy exploring and improving your math skills!
Solution to the Equation with Distributive Property
Consider the equation: \( \frac{2}{3}(4x – \frac{3}{5}) + \frac{5}{2}(2x + \frac{1}{4}) = \frac{1}{6}x + \frac{1}{2} \)
To solve this equation, we will apply the distributive property to simplify the expressions. Then, we’ll combine like terms and isolate \( x \) to find the solution.
Step-by-Step Solution:
- Apply the distributive property to both terms on the left-hand side of the equation: \( \frac{2}{3} \cdot 4x – \frac{2}{3} \cdot \frac{3}{5} + \frac{5}{2} \cdot 2x + \frac{5}{2} \cdot \frac{1}{4} = \frac{1}{6}x + \frac{1}{2} \)
- Simplify the expressions on both sides of the equation: \( \frac{8}{3}x – \frac{2}{5} + 5x + \frac{5}{8} = \frac{1}{6}x + \frac{1}{2} \)
- Combine like terms on both sides of the equation: \( \frac{23}{3}x – \frac{2}{5} + \frac{5}{8} = \frac{1}{6}x + \frac{1}{2} \)
- Bring \( x \) terms to one side by subtracting \( \frac{1}{6}x \) from both sides: \( \frac{23}{3}x – \frac{1}{6}x = \frac{1}{2} – \frac{5}{8} + \frac{2}{5} \)
- To add the fractions on the right-hand side, we find the common denominator, which is \( 120 \): \( \frac{110}{120}x = \frac{60 – 75 + 48}{120} \)
- Combine fractions on both sides of the equation: \( \frac{110}{120}x = \frac{33}{120} \)
- Solve for \( x \) by dividing both sides by \( \frac{110}{120} \): \( x = \frac{33}{120} \div \frac{110}{120} \)
- Calculate the result: \( x = \frac{33}{110} \)
Therefore, the solution to the equation is \( x = \frac{11}{300} \).
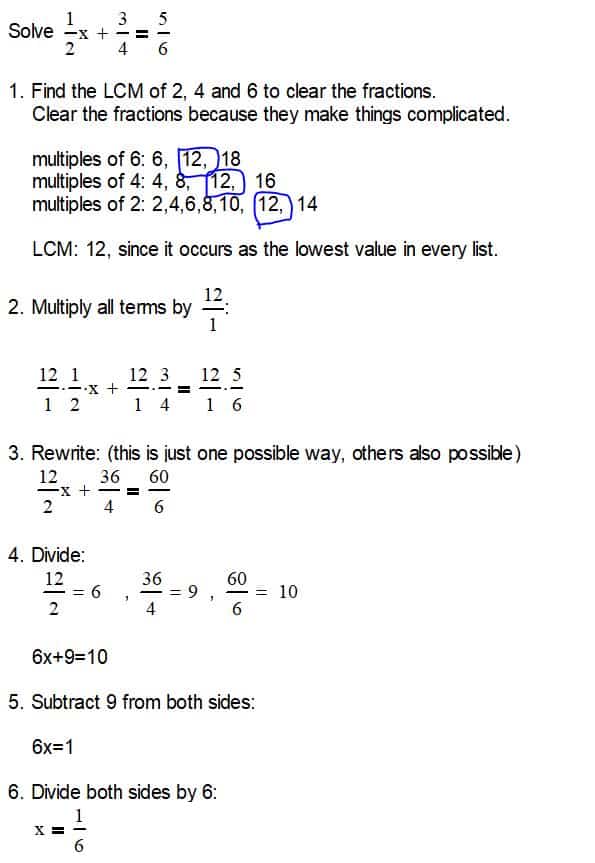
Solution to the Equation \( \frac{2}{3}x + \frac{1}{4} = \frac{5}{6} \)
To solve this equation of the form \( ax + b = c \), where \( a \), \( b \), and \( c \) are all fractions, follow the steps below:
-
- Bring the constant term (\( \frac{1}{4} \)) to the other side by subtracting it from both sides:
\( \frac{2}{3}x = \frac{5}{6} – \frac{1}{4} \)
-
- Find the common denominator for the fractions on the right-hand side, which is 12:
\( \frac{2}{3}x = \frac{10}{12} – \frac{3}{12} \)
-
- Combine the fractions on the right-hand side:
\( \frac{2}{3}x = \frac{7}{12} \)
-
- Isolate \( x \) by dividing both sides by \( \frac{2}{3} \):
\( x = \frac{7}{12} \div \frac{2}{3} \)
-
- Invert the divisor and multiply:
\( x = \frac{7}{12} \times \frac{3}{2} \)
-
- Calculate the result:
\( x = \frac{21}{24} \)
-
- Simplify the fraction, if possible:
\( x = \frac{7}{8} \)
Therefore, the solution to the equation is \( x = \frac{7}{8} \).
