Deriving the Power Series for \( e^{2x} \) from \( e^x \)

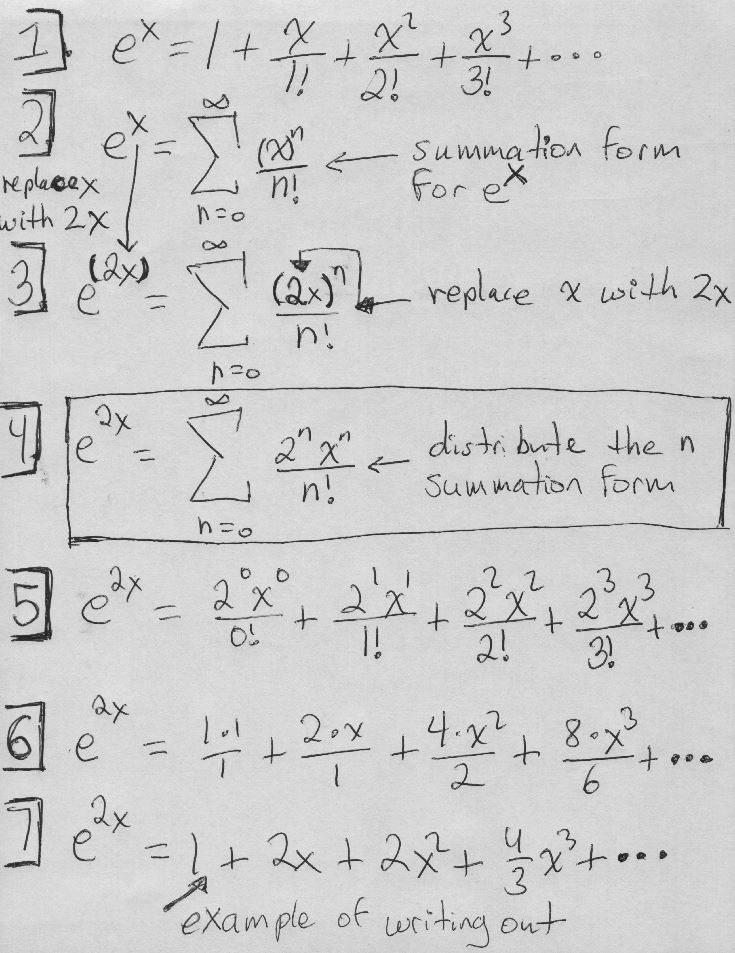
This guide explains how to derive the power series for \( e^{2x} \) starting from the Taylor series expansion of \( e^x \).

Step 1: Taylor Series for \( e^x \)

The Taylor series expansion for \( e^x \) is:

\[ e^x = \sum_{n=0}^{\infty} \frac{x^n}{n!} \]

Step 2: Substitute \( x \) with \( 2x \)

To find the series representation for \( e^{2x} \), we replace \( x \) with \( 2x \) in the above series:

\[ e^{2x} = \sum_{n=0}^{\infty} \frac{(2x)^n}{n!} \]

Step 3: Simplify

Simplifying, we get:

\[ e^{2x} = \sum_{n=0}^{\infty} \frac{2^n \cdot x^n}{n!} \]

Conclusion

By substituting \( x \) with \( 2x \) in the Taylor series expansion of \( e^x \), we have successfully derived the power series for \( e^{2x} \).

First Few Terms of the Power Series for \( e^{2x} \)

This guide provides the first few terms of the power series representation for \( e^{2x} \).

First Few Terms

The first few terms of the power series for \( e^{2x} \) are:

\[ 1 + 2x + \frac{4x^2}{2!} + \frac{8x^3}{3!} + \frac{16x^4}{4!} \]

Conclusion

These are the first few terms of the power series for \( e^{2x} \), which give us an approximation of the function near the origin.

Graph of \( e^{2x} \) and Its First Six Terms in the Power Series

This guide provides a graph that compares the function \( e^{2x} \) with its first six terms in the power series expansion.

Graph

The graph below shows \( e^{2x} \) and its first six terms \( 1 + 2x + \frac{4x^2}{2!} + \frac{8x^3}{3!} + \frac{16x^4}{4!} + \frac{32x^5}{5!} \) plotted from \( x = -2 \) to \( x = 2 \).

Conclusion

The graph provides a visual comparison between \( e^{2x} \) and its first six terms in the power series, helping to understand how well the series approximates the function near the origin.