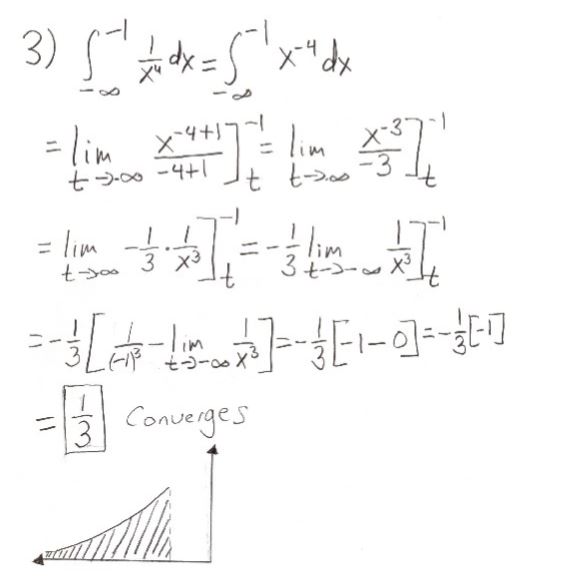
Detailed Walkthrough: Evaluating \( \int_{-\infty}^{-1} \frac{1}{x^4} \, dx \)

This article offers a comprehensive step-by-step guide on solving the improper integral \( \int_{-\infty}^{-1} \frac{1}{x^4} \, dx \). This integral is categorized as ‘improper’ due to its infinite lower limit, making it a crucial concept in advanced calculus and applications in fields like physics and engineering.

Step 1: Transforming to a Limit

Improper integrals involving infinite bounds can’t be evaluated directly. Therefore, we introduce a finite variable \( a \) that approaches the lower limit \(-\infty\). This allows us to transform the original integral into a limit-based form:

\[ \lim_{a \to -\infty} \int_{a}^{-1} \frac{1}{x^4} \, dx \]

Step 2: Antiderivative Identification

The next pivotal step is identifying the antiderivative of \( \frac{1}{x^4} \). This is the inverse operation of differentiation, allowing us to find a function whose derivative is \( \frac{1}{x^4} \). For this particular function, the antiderivative is:

\[ \int \frac{1}{x^4} \, dx = -\frac{1}{3x^3} + C \]

Step 3: Evaluating Between Bounds

With the antiderivative at hand, we substitute the upper and lower bounds into it. This gives us:

\[ -\frac{1}{3(-1)^3} – \left(-\frac{1}{3a^3}\right) = \frac{1}{3} + \frac{1}{3a^3} \]

Step 4: Applying the Limit

Our next move is to apply the limit to the above expression. This helps us understand the behavior of the integral as the lower limit approaches \(-\infty\):

\[ \lim_{a \to -\infty} \left( \frac{1}{3} + \frac{1}{3a^3} \right) \]

Step 5: Assessing Convergence or Divergence

The limit of \( \frac{1}{3a^3} \) as \( a \) approaches \(-\infty\) is zero. When summed with \( \frac{1}{3} \), the result remains \( \frac{1}{3} \).

Conclusion

This improper integral \( \int_{-\infty}^{-1} \frac{1}{x^4} \, dx \) is shown to converge to \( \frac{1}{3} \), which is a powerful testament to the capabilities of calculus in investigating mathematical phenomena extending to infinity.