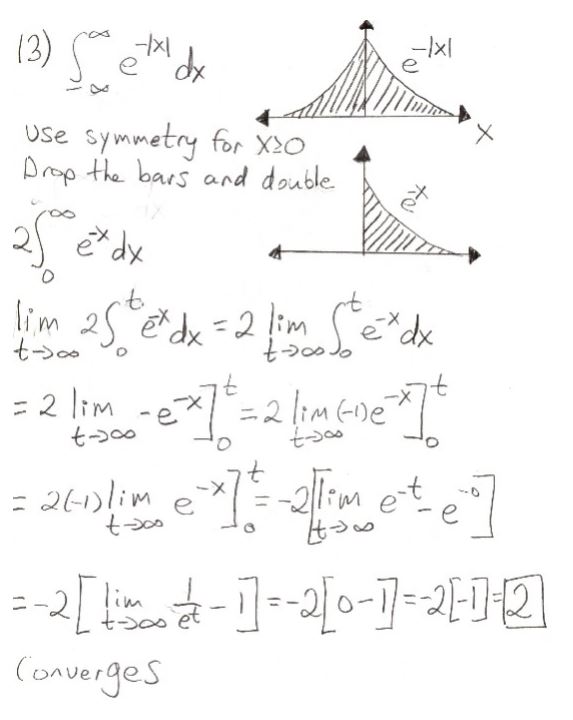
Step 1: Identifying the Function

The function we are interested in integrating is \( f(x) = e^{-|x|} \).

Step 2: Splitting the Integral

Since the function involves an absolute value, we split the integral into two parts:

\[ \int_{-\infty}^{0} e^x \, dx + \int_{0}^{\infty} e^{-x} \, dx \]

Step 3: Applying Limits

Both integrals are improper, so we use limits to evaluate them:

\[ \lim_{{a \to -\infty}} \int_{a}^{0} e^x \, dx + \lim_{{b \to \infty}} \int_{0}^{b} e^{-x} \, dx \]

Step 4: Evaluating the Integrals

The antiderivative of \( e^x \) is \( e^x \) and the antiderivative of \( e^{-x} \) is \( -e^{-x} \).

Step 5: Applying the Upper and Lower Limits

We apply the upper and lower limits to the antiderivatives:

\[ [e^x]_{a}^{0} + [-e^{-x}]_{0}^{b} \]

Step 6: Evaluating the Limits

After applying the limits, we find:

\[ (1 – 0) + (0 – (-1)) = 1 + 1 = 2 \]

Step 7: Final Result

The value of the integral is \( 2 \).