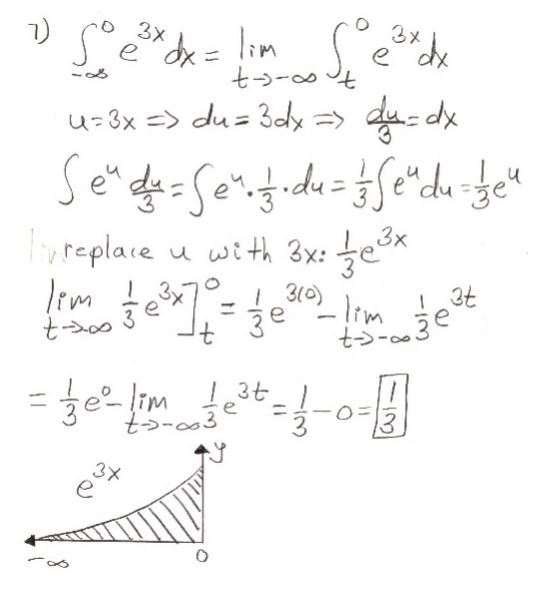
Step 1: Identifying the Function for Integration

We start by identifying the function we aim to integrate, which is \( e^{3x} \). This function is an exponential function with a base of \( e \) and an exponent of \( 3x \).

Step 2: Setting Up the Integral

We set up the integral with the given limits, which are \( x = -\infty \) and \( x = 0 \):

\[ \int_{-\infty}^{0} e^{3x} \, dx \]

Step 3: Applying Limits for the Improper Integral

Since the integral has an infinite lower limit, it is an improper integral. We use limits to evaluate it:

\[ \lim_{{a \to -\infty}} \int_{a}^{0} e^{3x} \, dx \]

Step 4: Finding the Antiderivative

The antiderivative of \( e^{3x} \) is \( \frac{1}{3} e^{3x} + C \), where \( C \) is the constant of integration.

Step 5: Applying the Upper and Lower Limits

We apply the upper and lower limits to the antiderivative:

\[ \left[\frac{1}{3} e^{3x}\right]_{a}^{0} \]

Step 6: Evaluating the Limits

After applying the limits, we find:

\[ \lim_{{a \to -\infty}} \left(\frac{1}{3} e^{3 \cdot 0} – \frac{1}{3} e^{3a}\right) = \frac{1}{3} – 0 = \frac{1}{3} \]

Step 7: Final Result and Interpretation

The integral converges to \( \frac{1}{3} \). This result is interesting because it shows that the function has a finite integral over the given range, highlighting its rapid decay as \( x \) approaches negative infinity.