1. Recognize the Integral: We start with the integral that we want to solve, which is \( \int e^{\sqrt{x}} dx \). This is not a standard form of integral that we can solve directly, so we need to use some techniques to simplify it.
2. Choose a Substitution: We notice that the exponent of \(e\) is \(\sqrt{x}\), which suggests that a substitution involving \(\sqrt{x}\) might simplify the integral. So, we let \(u = \sqrt{x}\). This implies that \(x = u^2\).
3. Find dx: To change the variable of integration from \(x\) to \(u\), we need to express \(dx\) in terms of \(du\). Differentiating \(x = u^2\) with respect to \(u\) gives \(dx = 2u du\).
4. Transform the Integral: We substitute \(u\) for \(\sqrt{x}\) and \(dx = 2u du\) into the integral. This transforms the integral into \(\int e^u 2u du\). This new integral is easier to solve, as it is now in the form of an integral of \(u e^u\), which we can solve using integration by parts.
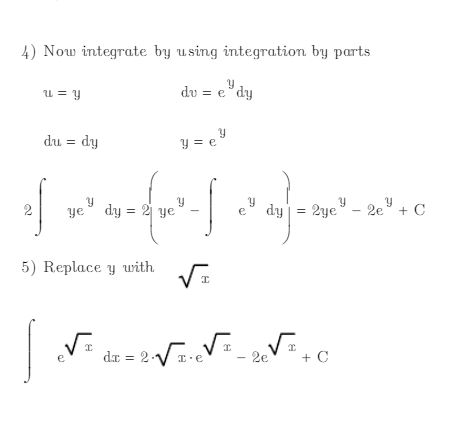
5. Integration by Parts: The method of integration by parts is given by the formula \(\int u dv = uv – \int v du\). We choose \(u = 2u\) and \(dv = e^u du\). Then \(du = 2 du\) and \(v = e^u\). Applying the formula, we get \(2u e^u – \int 2 e^u du\).
6. Solve the Simple Integral: The integral \(\int 2 e^u du\) is a standard integral, which is \(2e^u\). So, our original integral becomes \(2u e^u – 2e^u\).
7. Back Substitution: Now we substitute \(u = \sqrt{x}\) back into the integral, yielding \(2\sqrt{x} e^{\sqrt{x}} – 2e^{\sqrt{x}}\), which is the indefinite integral of \(e^{\sqrt{x}}\).
8. Add the Constant of Integration: The most general form of the integral includes a constant of integration, so the final answer is \(2\sqrt{x} e^{\sqrt{x}} – 2e^{\sqrt{x}} + C\), where \(C\) is the constant of integration. This represents the family of all antiderivatives of \(e^{\sqrt{x}}\).

Plot of \(e^{\sqrt{x}}\)

Plot of the Integral of \(e^{\sqrt{x}}\)