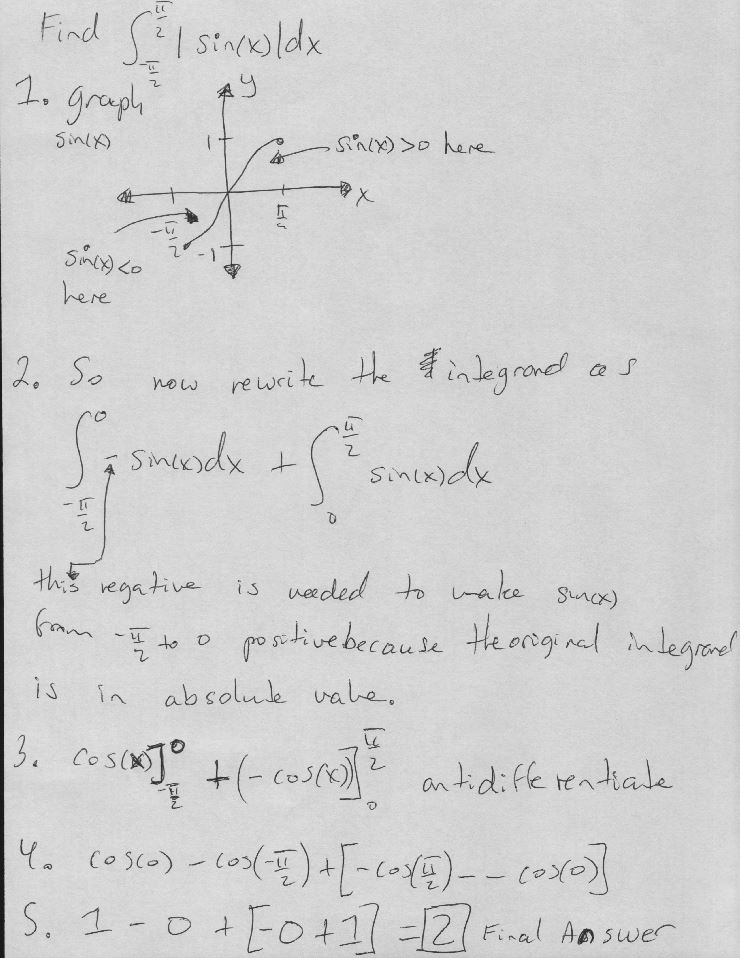
Evaluation of Integral of sin(x) from -π/2 to π/2

We aim to evaluate the following integral:

∫ from -π/2 to π/2 of sin(x) dx

Step 1: Find the Antiderivative

The antiderivative of sin(x) is -cos(x).

Step 2: Evaluate the Integral

We evaluate -cos(x) at the upper and lower limits and subtract:

-cos(π/2) + cos(-π/2) = 0 + 0 = 0

Final Result

The value of the integral ∫ from -π/2 to π/2 of sin(x) dx is 0.

Graphical Explanation for the Integral of sin(x) from -π/2 to π/2

We previously found that the integral:

∫ from -π/2 to π/2 of sin(x) dx = 0

Graphical Reasoning

The function sin(x) is symmetric about the y-axis. This means that for every positive value of sin(x) from 0 to π/2, there is a corresponding negative value from 0 to -π/2.

When you graph sin(x), you’ll notice that the area under the curve from 0 to π/2 is exactly the same as the area above the curve (or below the x-axis) from -π/2 to 0. These two areas cancel each other out, leading to a net area of zero.

Final Result

Graphically, it makes sense that the integral ∫ from -π/2 to π/2 of sin(x) dx is 0 because the positive and negative areas under the curve cancel each other out.