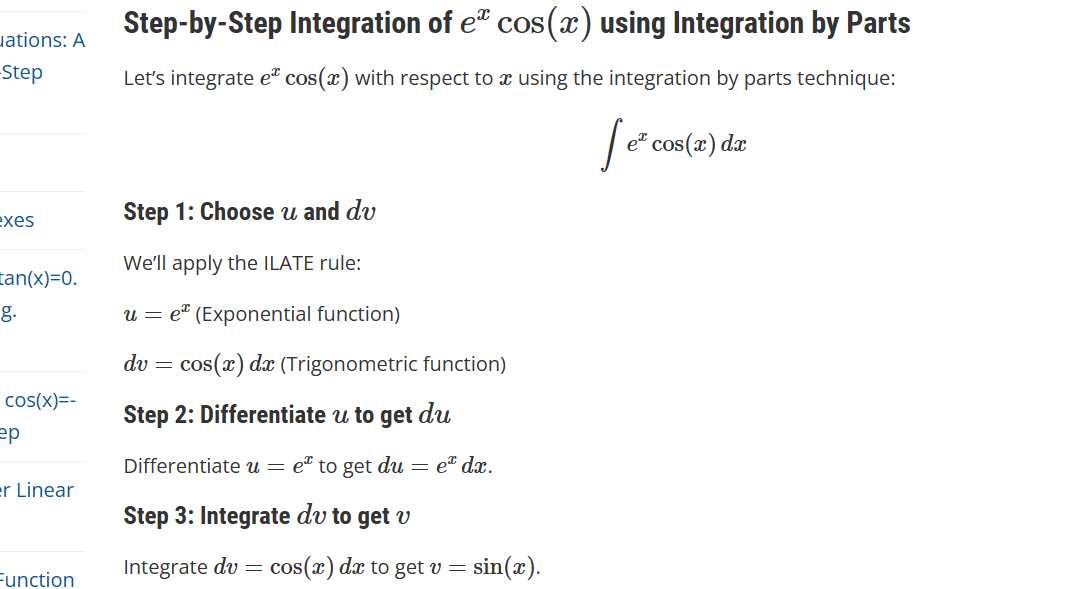
Understanding the ILATE Rule
The ILATE rule is a helpful mnemonic used to decide which parts of a product should be chosen as \(u\) and \(dv\) when applying the integration by parts technique. It stands for:
- I: Inverse Trigonometric Functions
- L: Logarithmic Functions
- A: Algebraic Functions (polynomials)
- T: Trigonometric Functions
- E: Exponential Functions
The rule suggests prioritizing differentiation over integration. Choose \(u\) from the ILATE order, and differentiate it to get \(du\). The remaining part becomes \(dv\), which should be integrated to get \(v\).
For example, in the integral of \(e^x \cos(x)\), we chose \(u = e^x\) and \(dv = \cos(x) \, dx\). This decision aligns with the ILATE rule’s preference for exponential functions over trigonometric functions.
Understanding the ILATE rule helps streamline the integration by parts process and leads to efficient solutions for integrals involving products of functions.
| Step | Expression | Explanation |
|---|---|---|
| 1 | ∫ eˣ cos(x) dx | Start with the original integral |
| 2 | u = eˣ, dv = cos(x) dx | Choose u and dv based on ILATE |
| 3 | du = eˣ dx | Differentiate u to get du |
| 4 | v = sin(x) | Integrate dv to get v |
| 5 | ∫ u dv = uv – ∫ v du | Apply the integration by parts formula |
| 6 | = eˣ sin(x) – ∫ eˣ sin(x) dx | Substitute u, du, and v into the formula |
| 7 | = eˣ sin(x) – (eˣ cos(x) – ∫ eˣ cos(x) dx) | Apply integration by parts to the new integral |
| 8 | 2I = eˣ sin(x) + eˣ cos(x) | Solve for the original integral I |
| 9 | I = 1/2 eˣ (sin(x) + cos(x)) + C | The integral is 1/2 eˣ (sin(x) + cos(x)) + C |