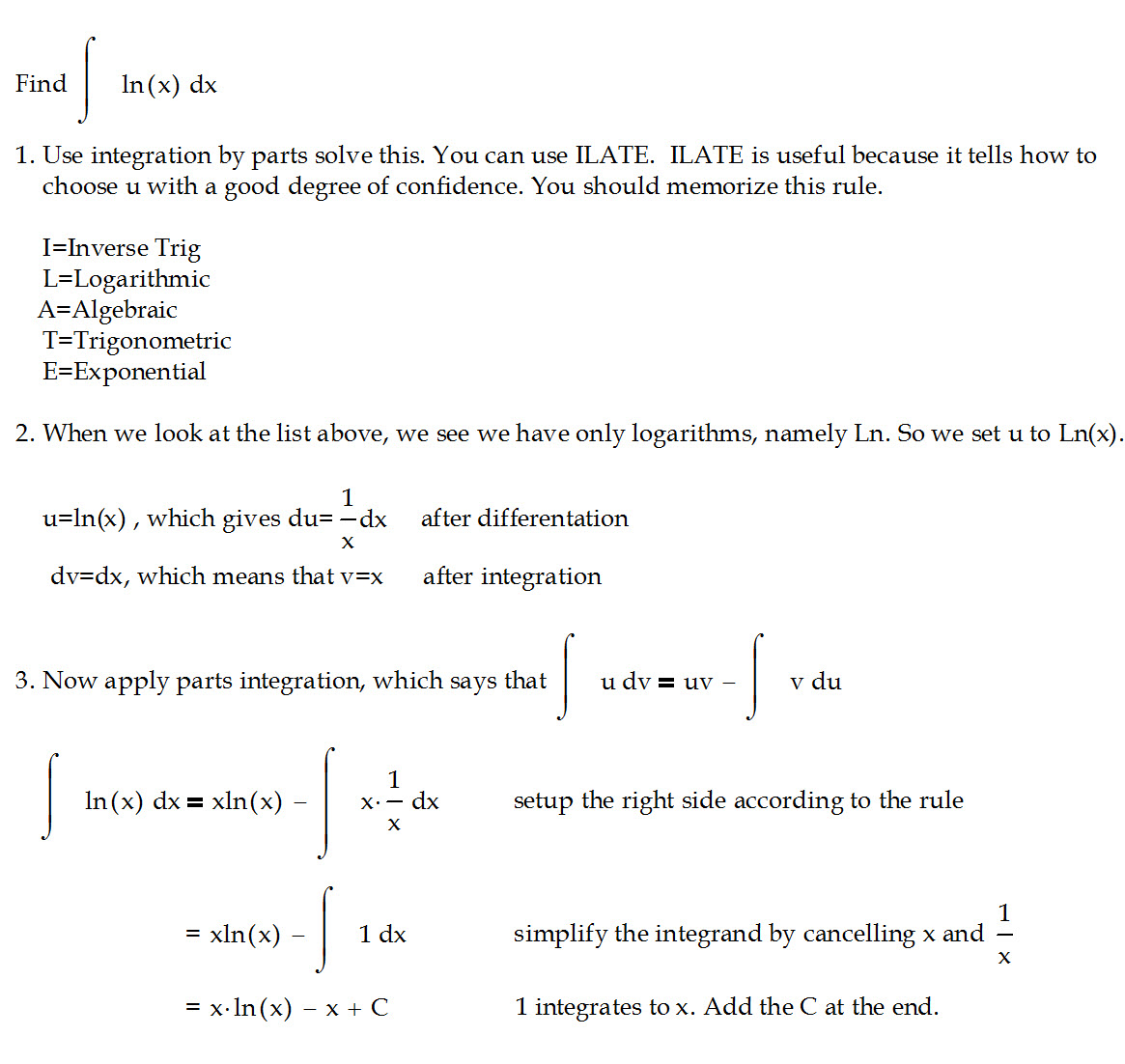
Introduction: Finding the antiderivative of the natural logarithm function, ln(x), is a captivating exploration in calculus. This guide will take you through the process using the ILATE method for integration by parts.

Step 1: Recognize the Integral

We want to find the integral of the natural logarithm function: ∫ ln(x) dx

Step 2: Apply Integration by Parts Using ILATE

Using the ILATE rule, we choose u = ln(x) and dv = dx. Then we find du = 1/x dx and v = x.

Step 3: Substitute into the Integration by Parts Formula

Substituting the chosen functions, we find: ∫ ln(x) dx = xln(x) – x + C

Conclusion: The antiderivative of ln(x) is xln(x) – x + C. This result showcases the elegance and complexity of calculus, and understanding it is a significant achievement in mathematical studies!