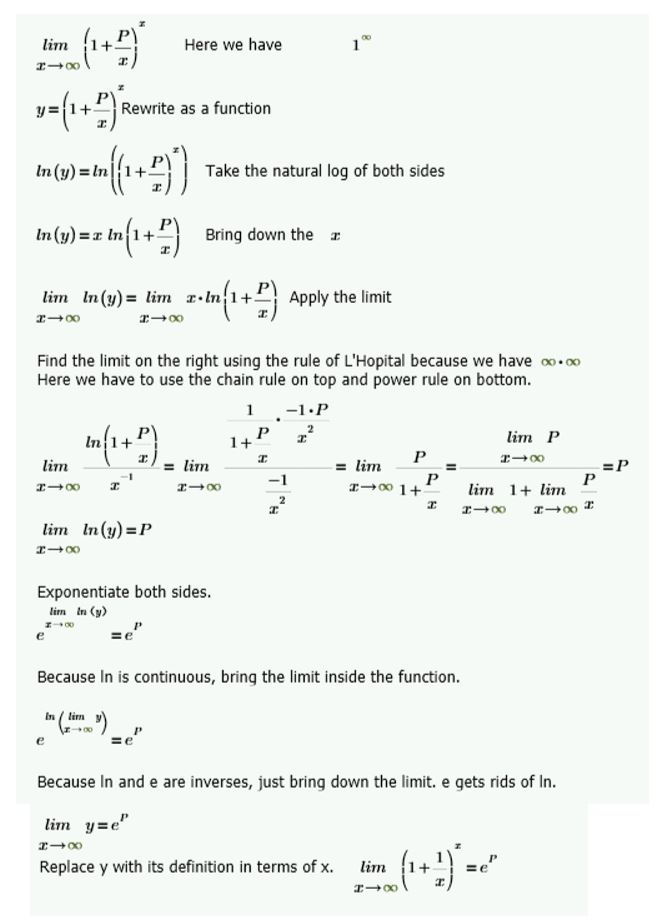
Detailed Steps for Calculating \( \lim_{{x \to \infty}} (1 + \frac{P}{x})^x \) Using \( x = e^{\ln(x)} \)

Step 1: Recognize the Complexity in the Limit

The limit \( \lim_{{x \to \infty}} (1 + \frac{P}{x})^x \) is complex due to the \( x \) in the exponent and the \( \frac{P}{x} \) term. Using \( x = e^{\ln(x)} \) can make it more manageable.

Step 2: Apply the Identity to \( x \)

We substitute \( x \) with \( e^{\ln(x)} \) in the exponent, keeping the base \( (1 + \frac{P}{x}) \) the same: \[ (1 + \frac{P}{x})^{e^{\ln(x)}} \]

Step 3: Simplify the Exponent Using Natural Logarithm Properties

Using the property \( a^{m^n} = (a^m)^n \), the expression becomes: \[ \left( (1 + \frac{P}{x})^{\ln(x)} \right)^{e} \]

Step 4: Tackle the Inner Limit \( \lim_{{x \to \infty}} (1 + \frac{P}{x})^{\ln(x)} \)

Now, we need to find the limit of \( (1 + \frac{P}{x})^{\ln(x)} \) as \( x \to \infty \). Using techniques such as L’Hôpital’s Rule or series expansion, let’s say this limit is \( L \).

Step 5: Insert the Inner Limit Result into the Original Limit

Having found \( L \), we then have: \[ \left( L \right)^{e} = L \times L \times \cdots \text{(e times)} = L^e \] as the overall limit.

Step 6: Summary

Using the identity \( x = e^{\ln(x)} \), we’ve successfully simplified and solved the limit \( \lim_{{x \to \infty}} (1 + \frac{P}{x})^x \) to be \( L^e \), where \( L \) is the inner limit we calculated.