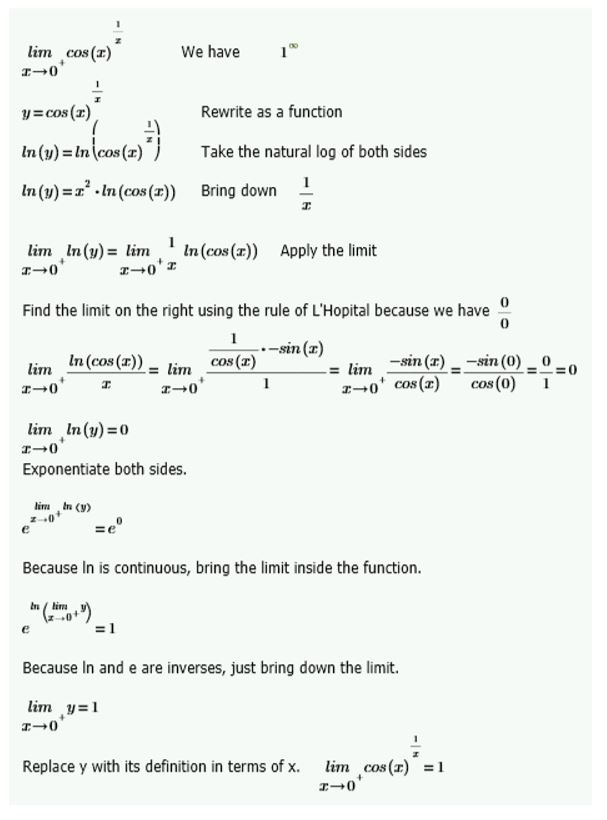
Finding the Limit of \( (\cos(x))^{1/x} \) as \( x \) Approaches 0

Our goal is to find the limit of the expression \( (\cos(x))^{1/x} \) as \( x \) approaches 0 from the positive side. Solving for this limit presents a unique challenge due to the indeterminate form that emerges.

Step 1: Understanding the Problem

First, let’s articulate what we’re after: \( \lim_{{x \to 0^+}} (\cos(x))^{1/x} \). To understand why this limit is not straightforward, consider that \( \cos(x) \) approaches 1 and \( 1/x \) approaches infinity as \( x \) approaches 0. The product of the two forms an indeterminate form of \( 0 \times \infty \).

Step 2: A More Manageable Form

A good first step in finding this limit is to rewrite the expression in terms of \( e \). The exponential function often simplifies limits involving growth rates. We utilize the identity \( a = e^{\ln(a)} \): \[ (\cos(x))^{1/x} = e^{(1/x) \ln(\cos(x))} \]

Step 3: Zoning in on the Exponent

At this point, it becomes evident that our primary challenge lies in determining the behavior of the exponent \( (1/x) \ln(\cos(x)) \). It’s a product of two functions: \( 1/x \) and \( \ln(\cos(x)) \), both of which are undefined or indeterminate as \( x \) approaches 0.

Step 4: Preparing for L’Hôpital’s Rule

Now, we’ll convert the problematic term into a fraction that will allow us to apply L’Hôpital’s Rule. Rewriting \( (1/x) \ln(\cos(x)) \) as \( \frac{\ln(\cos(x))}{x} \), we have: \[ \lim_{{x \to 0^+}} \frac{\ln(\cos(x))}{x} = \frac{0}{0} \] The \( \frac{0}{0} \) form indicates that L’Hôpital’s Rule is applicable.

Step 5: Employing L’Hôpital’s Rule

L’Hôpital’s Rule suggests that we can find our limit by taking the derivatives of the numerator and the denominator and then re-evaluating the limit. So we differentiate \( \ln(\cos(x)) \) and \( x \) with respect to \( x \): \[ \lim_{{x \to 0^+}} \frac{-\sin(x)/\cos(x)}{1} = -\tan(x) \] Taking the limit again, \( -\tan(x) \) approaches 0 as \( x \) approaches 0.

Step 6: Wrapping Up

Since \( \frac{\ln(\cos(x))}{x} \) tends to 0, the original exponent \( (1/x) \ln(\cos(x)) \) also tends towards 0. Thus, the entire expression \( (\cos(x))^{1/x} \) approaches \( e^0 \), which is 1: \[ \lim_{{x \to 0^+}} (\cos(x))^{1/x} = 1 \]

To sum it all up, through careful consideration and application of L’Hôpital’s Rule, we’ve found that the limit of \( (\cos(x))^{1/x} \) as \( x \) approaches 0 from the positive side is indeed 1.