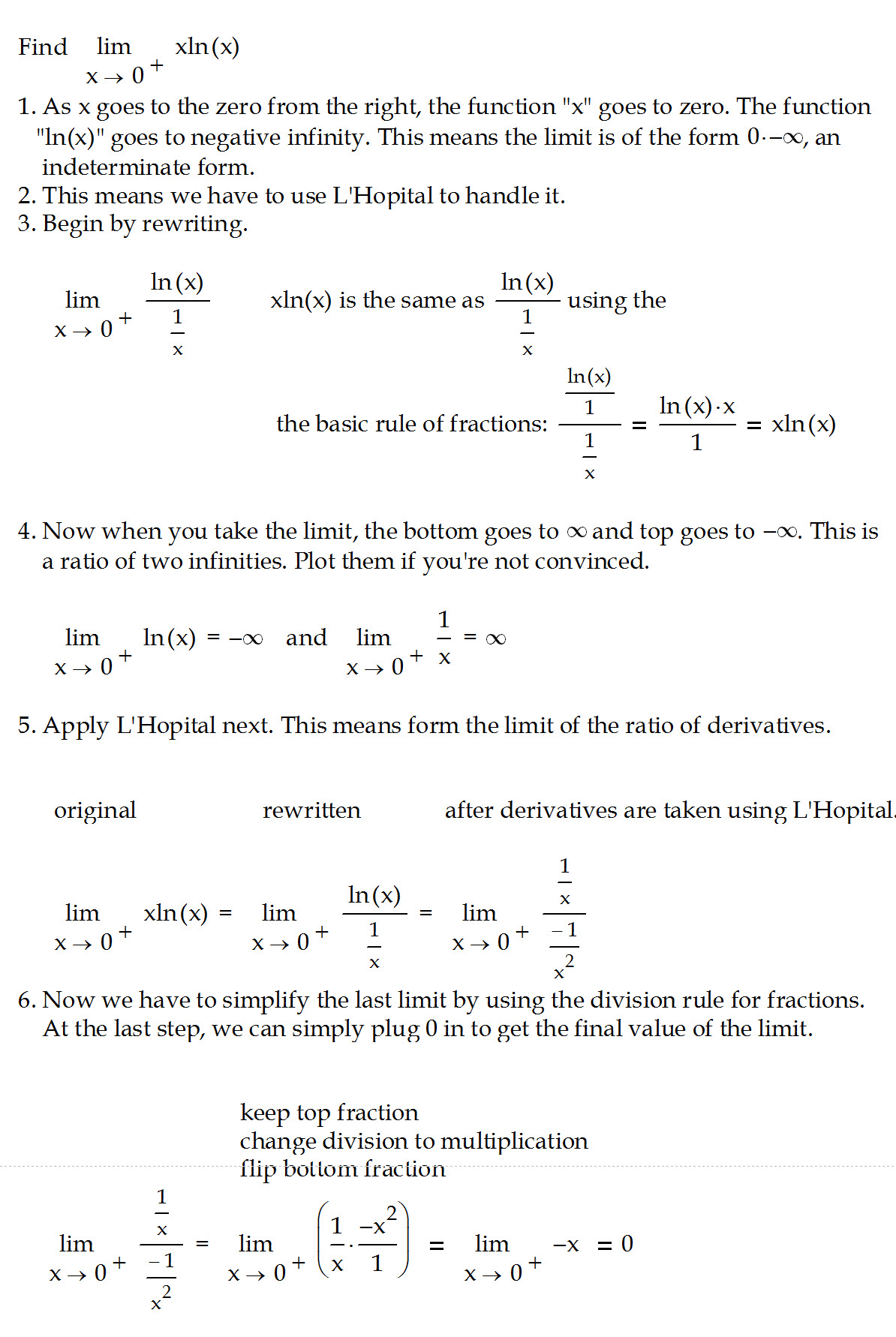
Original Question

Find the limit of the function f(x) = x ln(x) as x approaches 0 from the right.

Step 1: Recognize the Indeterminate Form

As x approaches 0, the expression x ln(x) takes the indeterminate form 0 ⋅ (-∞). We need to rewrite it in a form where L’Hôpital’s Rule can be applied.

Step 2: Rewrite the Expression as a Fraction

We can rewrite the expression as a fraction by considering it as a ratio of two functions:

\[ \frac{{\ln(x)}}{{1/x}} \]

Step 3: Check the Conditions for L’Hôpital’s Rule

As x approaches 0 from the right, both the numerator and denominator approach -∞, so we have an indeterminate form of the type \(\frac{{-\infty}}{{-\infty}}\), and L’Hôpital’s Rule can be applied.

Step 4: Apply L’Hôpital’s Rule

We take the derivative of the numerator and the derivative of the denominator:

– Derivative of the numerator: d/dx ln(x) = 1/x

– Derivative of the denominator: d/dx 1/x = -1/x²

Now, we find the limit of the ratio of these derivatives:

\[ \lim_{{x \to 0^+}} \frac{{1/x}}{{-1/x²}} = \lim_{{x \to 0^+}} -x²/x = \lim_{{x \to 0^+}} -x = 0 \]

Conclusion

The limit of the function f(x) = x ln(x) as x approaches 0 from the right is 0.

Summary

\[ \lim_{{x \to 0^+}} x ln(x) = \lim_{{x \to 0^+}} \frac{{\ln(x)}}{{1/x}} = \lim_{{x \to 0^+}} \frac{{1/x}}{{-1/x²}} = \lim_{{x \to 0^+}} -x²/x = \lim_{{x \to 0^+}} -x = 0 \]