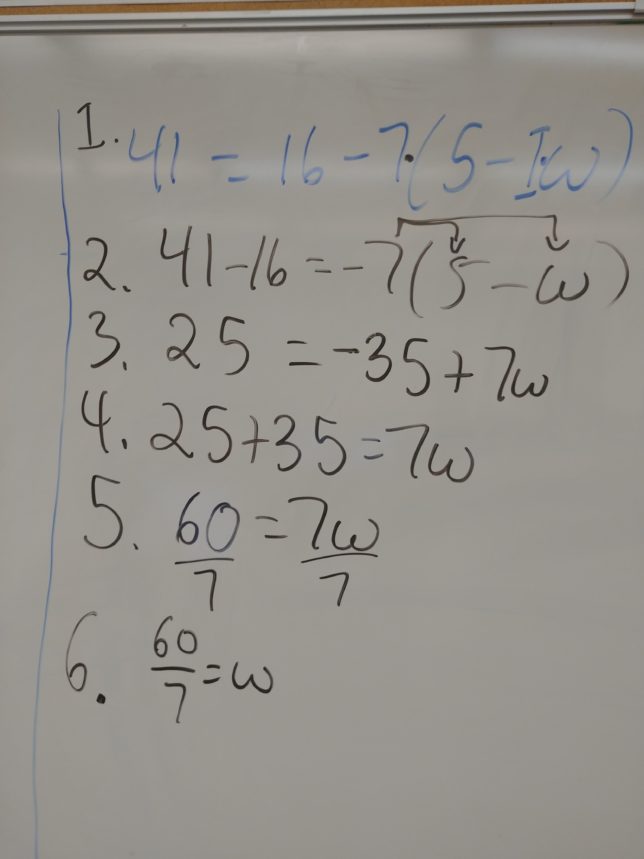41 = 16 – 7(5 – w) → Starting equation
41 = 16 – 7(5) + 7(w) → Distribute -7 to both terms inside the parentheses
41 = 16 – 35 + 7w → Calculate -7 multiplied by 5 to get -35 and 7 multiplied by w to get 7w
41 = -19 + 7w → Combine like terms (16 – 35 = -19)
41 + 19 = 7w → Add 19 to both sides to isolate 7w
60 = 7w → Simplify (41 + 19 = 60)
60 / 7 = w → Divide both sides by 7 to solve for w
w = 60 / 7 → Final answer for w
27 = 11 – 3(6 – z) → Starting equation
27 = 11 – 3 × 6 + 3 × z → Distribute -3 to both terms inside the parentheses
27 = 11 – 18 + 3z → Calculate -3 multiplied by 6 to get -18 and 3 multiplied by z to get 3z
27 = -7 + 3z → Combine like terms (11 – 18 = -7)
27 + 7 = 3z → Add 7 to both sides to isolate 3z
34 = 3z → Simplify (27 + 7 = 34)
34 / 3 = z → Divide both sides by 3 to solve for z
z = 34 / 3 → Final answer for z