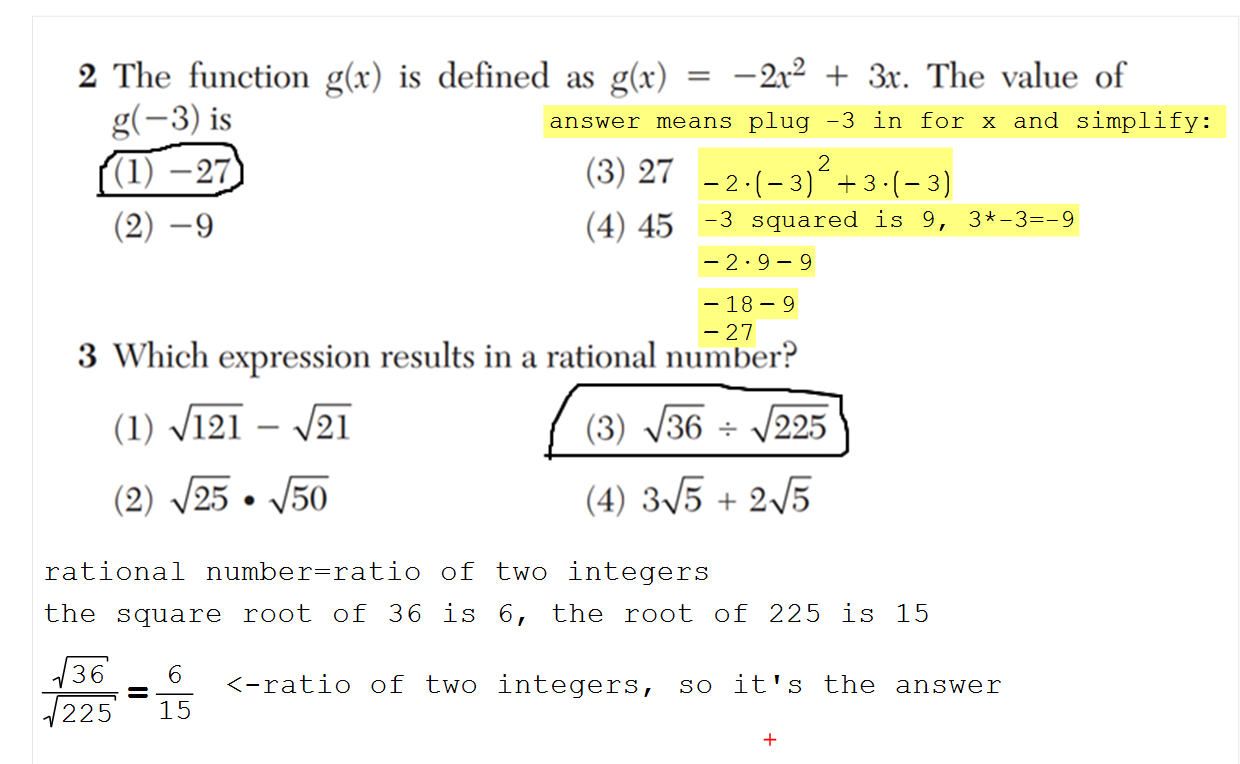
Evaluate the function g(x) = -2x² + 3x at x = -3.
Let x = -3
(-3) × (-3) = 9
-2 × 9 = -18
3 × (-3) = -9
-18 – 9 = -27
g(-3) = -27
Summary: g(-3) = -2 × (-3)² + 3 × (-3) = -2 × 9 – 9 = -18 – 9 = -27
Evaluate the trinomial function f(x) = 2x³ – 3x² + 4x at x = 2.
Let’s start by defining the variable x:
Let x = 2
Now, calculate the cube of x:
2³ = 2 × 2 × 2 = 8
Multiply 2 by the cube of x:
2 × 8 = 16
Calculate the square of x:
2² = 2 × 2 = 4
Multiply -3 by the square of x:
-3 × 4 = -12
Multiply 4 by x:
4 × 2 = 8
Add the three results together to find the value of f(x):
16 – 12 + 8 = 12
Final result:
f(2) = 12
Summary: f(2) = 2 × 2³ – 3 × 2² + 4 × 2 = 2 × 8 – 3 × 4 + 8 = 16 – 12 + 8 = 12
Evaluate the trinomial function h(x) = 3x³ – 5x² + 7 at x = 1.
Let’s start by defining the variable x:
Let x = 1
Now, calculate the cube of x:
1³ = 1 × 1 × 1 = 1
Multiply 3 by the cube of x:
3 × 1 = 3
Calculate the square of x:
1² = 1 × 1 = 1
Multiply -5 by the square of x:
-5 × 1 = -5
Add the constant term:
7
Add the three results together to find the value of h(x):
3 – 5 + 7 = 5
Final result:
h(1) = 5
Summary: h(1) = 3 × 1³ – 5 × 1² + 7 = 3 × 1 – 5 × 1 + 7 = 3 – 5 + 7 = 5
Evaluate the trinomial function k(x) = -4x³ + 2x² – 6 at x = -2.
Note: When inputting negative numbers in calculations, it’s important to use parentheses to ensure the correct order of operations and avoid errors.
Let’s start by defining the variable x:
Let x = -2
Now, calculate the cube of x (using parentheses):
(-2)³ = (-2) × (-2) × (-2) = -8
Multiply -4 by the cube of x:
-4 × (-8) = 32
Calculate the square of x (using parentheses):
(-2)² = (-2) × (-2) = 4
Multiply 2 by the square of x:
2 × 4 = 8
Add the constant term:
-6
Add the three results together to find the value of k(-2):
32 + 8 – 6 = 34
Final result:
k(-2) = 34
Summary: k(-2) = -4 × (-2)³ + 2 × (-2)² – 6 = -4 × (-8) + 2 × 4 – 6 = 32 + 8 – 6 = 34
