Discover the Geometry of Multivariable Functions: Sketching the Plane Represented by f(x,y) = 4 – 2x – y

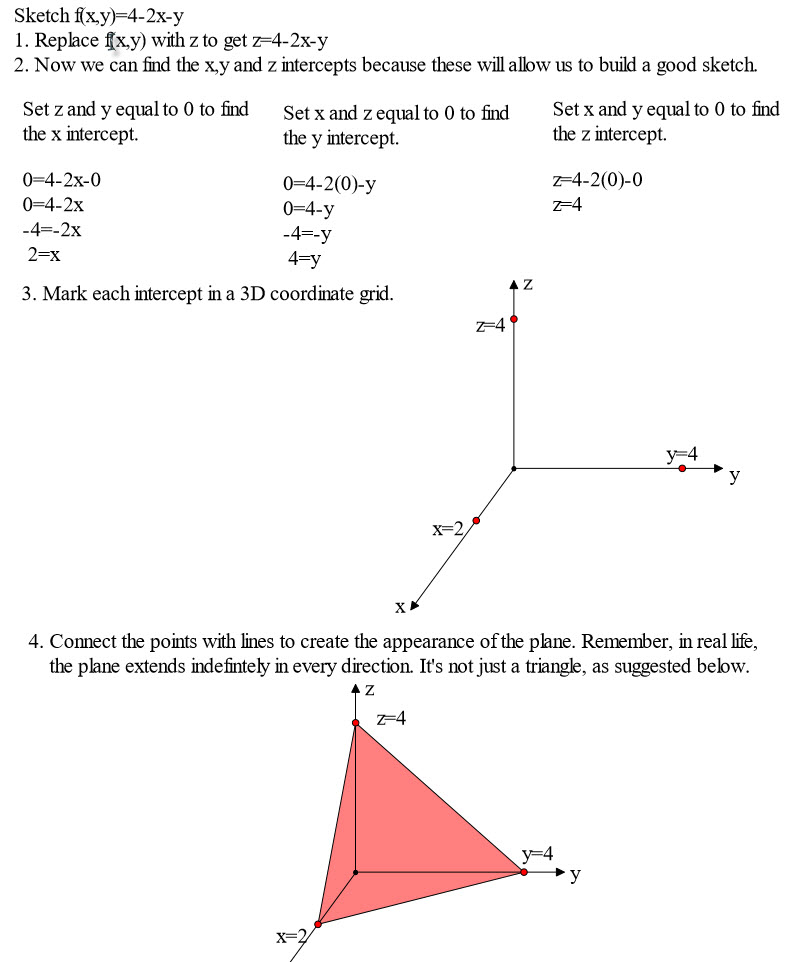
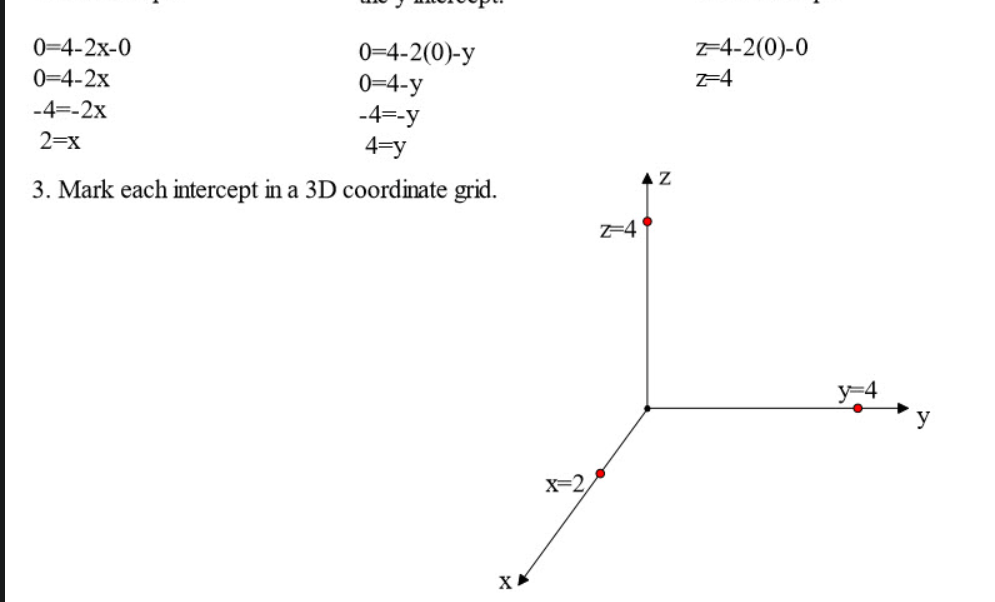
This plane intersects the x-axis at (2, 0, 0), the y-axis at (0, 4, 0), and reaches a height of 4 at the origin. Sloping downward as x and y increase, it forms a vivid geometric shape in three-dimensional space, illustrating the rich interplay between algebra and geometry.

Steps to Sketch the Plane:

• Identify the Intercepts: x-intercept at (2, 0, 0), y-intercept at (0, 4, 0), z-intercept at (0, 0, 4).
• Draw the Lines Connecting the Intercepts.
• Fill in the Plane, extending the triangle formed by the intercepts.
• Observe the Orientation, noticing the downward slope as x and y increase.
• Optional – Add Contour Lines to represent levels of the function, such as the line where f(x,y) = 2.