Explore the fascinating world of limits in calculus with our comprehensive guide. We delve into three distinct examples to help you understand this essential concept: finding the limit of a function with a hole in both simple and complex scenarios, and evaluating the limit of a piecewise function made of two linear parts. Whether you’re just starting your calculus journey or looking to deepen your understanding, our step-by-step explanations provide valuable insights into the behavior of functions as they approach specific points. Dive in to master the art of finding limits!
| \( f(x) = \frac{{(x – 2)(x + 3)}}{{(x – 2)}} \) | This is the given function. Notice that there is a common factor in the numerator and denominator, which creates a hole at \( x = 2 \). |
| \( f(x) = x + 3 \) | We can simplify the function by canceling the common factor \( (x – 2) \) from the numerator and denominator. This leaves us with the simplified function \( f(x) = x + 3 \), which is valid for all \( x \neq 2 \). |
| \( \lim_{{x \to 2}} (x + 3) = 5 \) | Now, we can find the limit as \( x \) approaches 2 by substituting \( x = 2 \) into the simplified function. The limit is 5. |
The limit of the function \( f(x) = \frac{{(x – 2)(x + 3)}}{{(x – 2)}} \) as \( x \) approaches 2 is 5, even though there is a hole at \( x = 2 \). This example illustrates how to find the limit of a function at a point where there is a removable discontinuity or a hole.
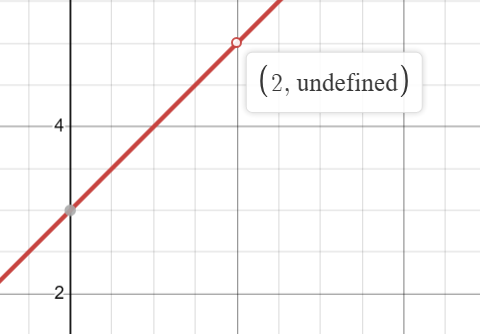
Finding the Limit of a Function with a Hole: A Comprehensive Guide
In this guide, we will explore how to find the limit of a function that has a hole, using a more advanced example. Understanding the concept of limits, especially when dealing with holes or removable discontinuities, is fundamental in calculus.
| \( f(x) = \frac{{(x^2 – 4)(x + 2)}}{{(x – 2)}} \) | This is our given function. Notice the common factor \( (x + 2) \) in the numerator and denominator, which creates a hole at \( x = -2 \). |
| \( f(x) = x^2 – 4 \) | We simplify the function by canceling the common factor \( (x + 2) \) from the numerator and denominator. This leaves us with the simplified function \( f(x) = x^2 – 4 \), which is valid for all \( x \neq -2 \). |
| \( \lim_{{x \to -2}} (x^2 – 4) = 0 \) | We find the limit as \( x \) approaches -2 by substituting \( x = -2 \) into the simplified function. The limit is 0. |
The limit of the function \( f(x) = \frac{{(x^2 – 4)(x + 2)}}{{(x – 2)}} \) as \( x \) approaches -2 is 0, even though there is a hole at \( x = -2 \). This example demonstrates the process of finding the limit at a point of removable discontinuity in a more complex function.
If you’re studying calculus and want to learn more about limits, continuity, and other essential concepts, explore our comprehensive resources and tutorials.

Finding the Limit of a Piecewise Function: A Detailed Guide
In this tutorial, we will learn how to find the limit of a piecewise function composed of two linear functions. Piecewise functions are common in mathematics and present unique challenges when finding limits.
Consider the piecewise function:
\[ f(x) = \begin{cases} 2x + 3 & \text{if } x < 1 \\ -x + 5 & \text{if } x \geq 1 \end{cases} \]
| \( \lim_{{x \to 1^-}} (2x + 3) = 5 \) | We find the left-hand limit as \( x \) approaches 1 by substituting \( x = 1 \) into the first linear function. The left-hand limit is 5. |
| \( \lim_{{x \to 1^+}} (-x + 5) = 4 \) | We find the right-hand limit as \( x \) approaches 1 by substituting \( x = 1 \) into the second linear function. The right-hand limit is 4. |
| \( \lim_{{x \to 1}} f(x) \) | The limit as \( x \) approaches 1 does not exist because the left-hand limit and right-hand limit are not equal. |
The limit of the given piecewise function as \( x \) approaches 1 does not exist. This example illustrates the importance of evaluating both the left-hand and right-hand limits when dealing with piecewise functions.
If you’re interested in learning more about limits, continuity, and other essential calculus concepts, explore our in-depth resources and tutorials.
