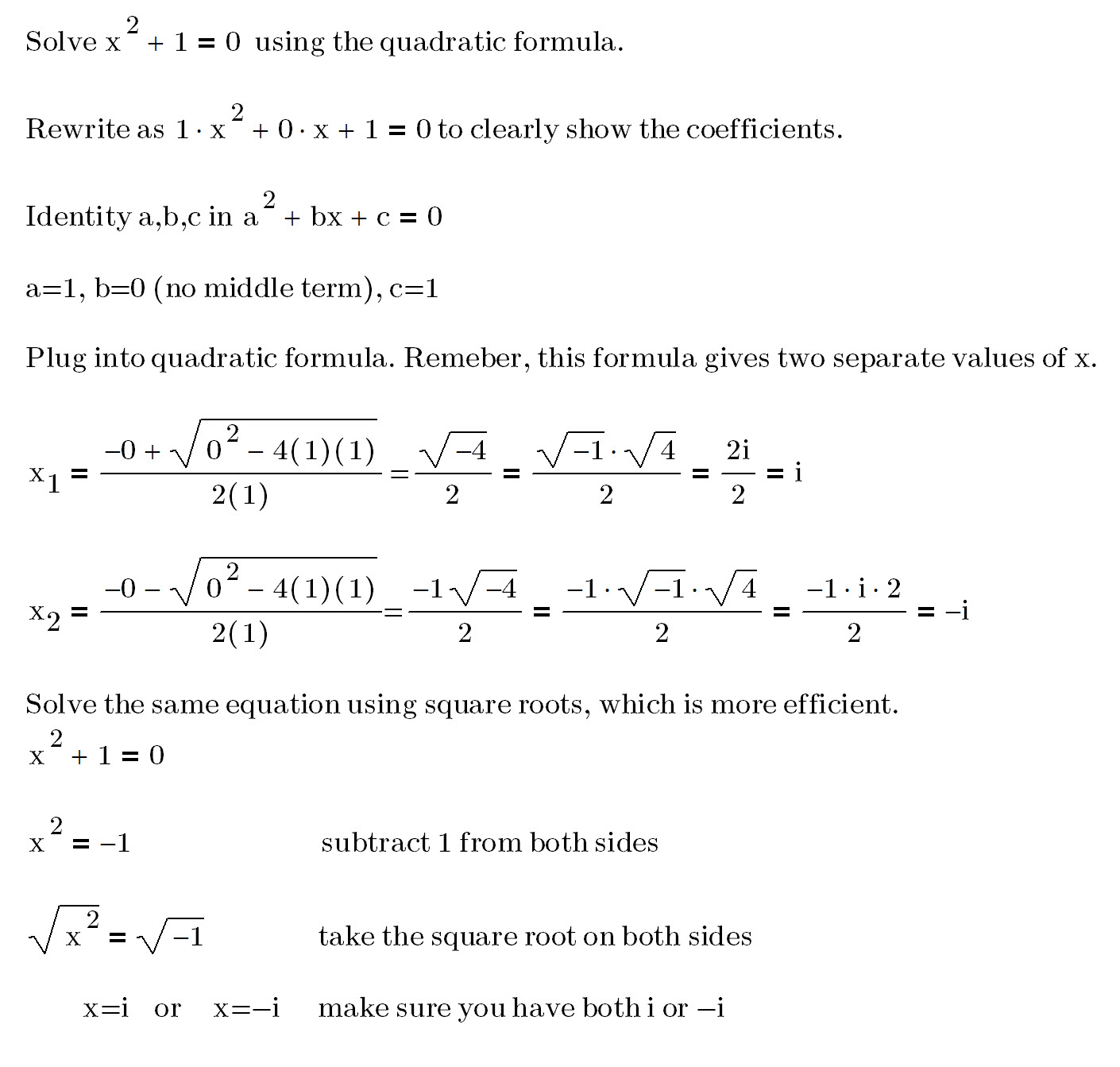
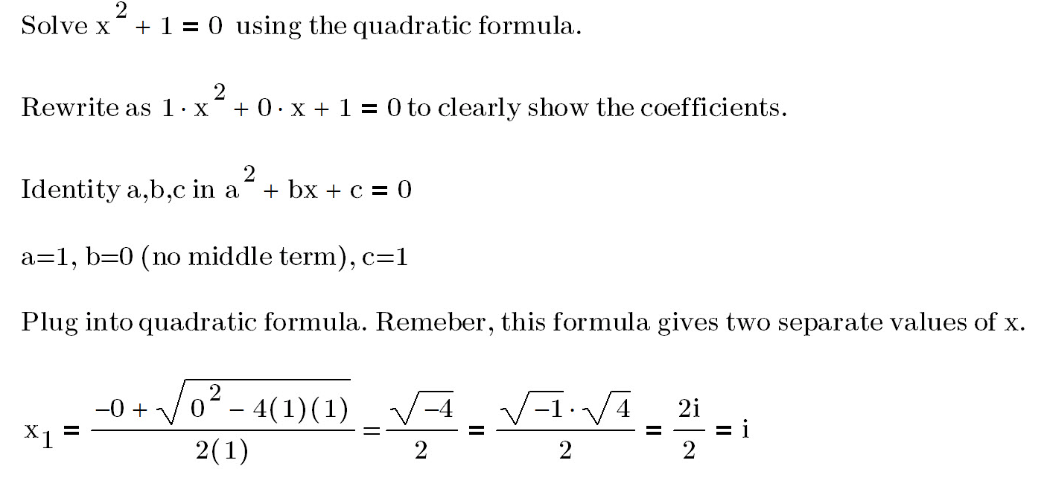
Solving the Quadratic Equation x² + 1 = 0: A Step-by-Step Guide

Step 1: Identify the Coefficients

Math: a = 1, b = 0, c = 1 | Explanation: Coefficients for the standard form ax² + bx + c = 0.

Step 2: Calculate the Discriminant

Math: D = b² – 4ac = 0 – 4 = -4 | Explanation: Calculating the discriminant.

Step 3: Analyze the Discriminant

Explanation: A negative discriminant indicates complex solutions.

Step 4: Apply the Quadratic Formula

Math: x = (-b ± √(b² – 4ac)) / 2a = (0 ± √(-4)) / 2 = (0 ± 2i) / 2 = 0 ± i | Explanation: Applying the quadratic formula to find the complex solutions.

Conclusion

The solutions to the quadratic equation x² + 1 = 0 are x = i and x = -i. This step-by-step guide offers valuable insights into solving quadratic equations, a fundamental concept in algebra. Explore more about this specific example and other mathematical concepts with this comprehensive guide.