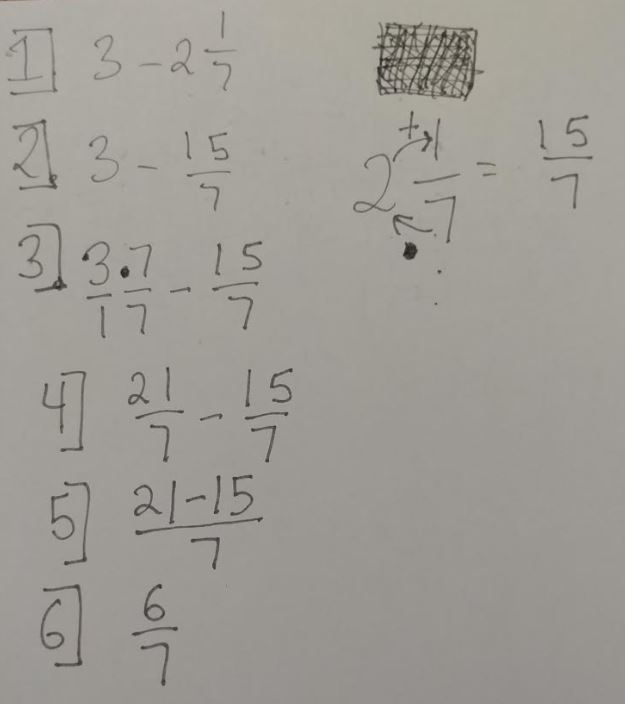Subtracting a Mixed Number from a Whole Number: Detailed Guide
Example: Let’s subtract the mixed number \(2\frac{3}{4}\) from the whole number \(7\).
Step 1: Write down the numbers: \(7 – 2\frac{3}{4}\).
Step 2: Convert the mixed number to an improper fraction. Multiply the whole number part by the denominator and add the numerator: \(2 \times 4 + 3 = 11\), so \(2\frac{3}{4} = \frac{11}{4}\).
Step 3: Convert the whole number to a fraction with the same denominator as the mixed number. Multiply \(7\) by \(4\): \(7 \times \frac{4}{4} = \frac{28}{4}\).
Step 4: Subtract the fractions by subtracting the numerators and keeping the same denominator: \(\frac{28}{4} – \frac{11}{4} = \frac{28 – 11}{4} = \frac{17}{4}\).
Step 5: Convert the result back to a mixed number. Divide the numerator by the denominator: \(17 \div 4 = 4\) with a remainder of \(1\), so \(\frac{17}{4} = 4\frac{1}{4}\).
Conclusion: The result of subtracting the mixed number \(2\frac{3}{4}\) from the whole number \(7\) is \(4\frac{1}{4}\). This process involves several steps, including converting the mixed number to an improper fraction, aligning the denominators, performing the subtraction, and converting back to a mixed number. Each step is broken down into smaller parts to make the process easier to understand.
Subtracting a Mixed Number from a Whole Number: Another Detailed Guide
Example: Let’s subtract the mixed number \(3\frac{2}{5}\) from the whole number \(10\).
Step 1: Write down the numbers: \(10 – 3\frac{2}{5}\).
Step 2: Convert the mixed number to an improper fraction. Multiply the whole number part by the denominator and add the numerator: \(3 \times 5 + 2 = 17\), so \(3\frac{2}{5} = \frac{17}{5}\).
Step 3: Convert the whole number to a fraction with the same denominator as the mixed number. Multiply \(10\) by \(5\): \(10 \times \frac{5}{5} = \frac{50}{5}\).
Step 4: Subtract the fractions by subtracting the numerators and keeping the same denominator: \(\frac{50}{5} – \frac{17}{5} = \frac{50 – 17}{5} = \frac{33}{5}\).
Step 5: Convert the result back to a mixed number. Divide the numerator by the denominator: \(33 \div 5 = 6\) with a remainder of \(3\), so \(\frac{33}{5} = 6\frac{3}{5}\).
Conclusion: The result of subtracting the mixed number \(3\frac{2}{5}\) from the whole number \(10\) is \(6\frac{3}{5}\). This step-by-step guide demonstrates how to convert mixed numbers to improper fractions, align denominators, perform subtraction, and convert back to mixed numbers, providing a comprehensive understanding of the process.
Subtracting a Mixed Number from a Whole Number: A Comprehensive Guide
Example: Let’s subtract the mixed number \(4\frac{3}{7}\) from the whole number \(15\).
Step 1: Write down the numbers: \(15 – 4\frac{3}{7}\).
Step 2: Convert the mixed number to an improper fraction. Multiply the whole number part by the denominator and add the numerator: \(4 \times 7 + 3 = 31\), so \(4\frac{3}{7} = \frac{31}{7}\).
Step 3: Convert the whole number to a fraction with the same denominator as the mixed number. Multiply \(15\) by \(7\): \(15 \times \frac{7}{7} = \frac{105}{7}\).
Step 4: Subtract the fractions by subtracting the numerators and keeping the same denominator: \(\frac{105}{7} – \frac{31}{7} = \frac{105 – 31}{7} = \frac{74}{7}\).
Step 5: Convert the result back to a mixed number. Divide the numerator by the denominator: \(74 \div 7 = 10\) with a remainder of \(4\), so \(\frac{74}{7} = 10\frac{4}{7}\).
Conclusion: The result of subtracting the mixed number \(4\frac{3}{7}\) from the whole number \(15\) is \(10\frac{4}{7}\). This step-by-step guide demonstrates how to convert mixed numbers to improper fractions, align denominators, perform subtraction, and convert back to mixed numbers, providing a comprehensive understanding of the process.