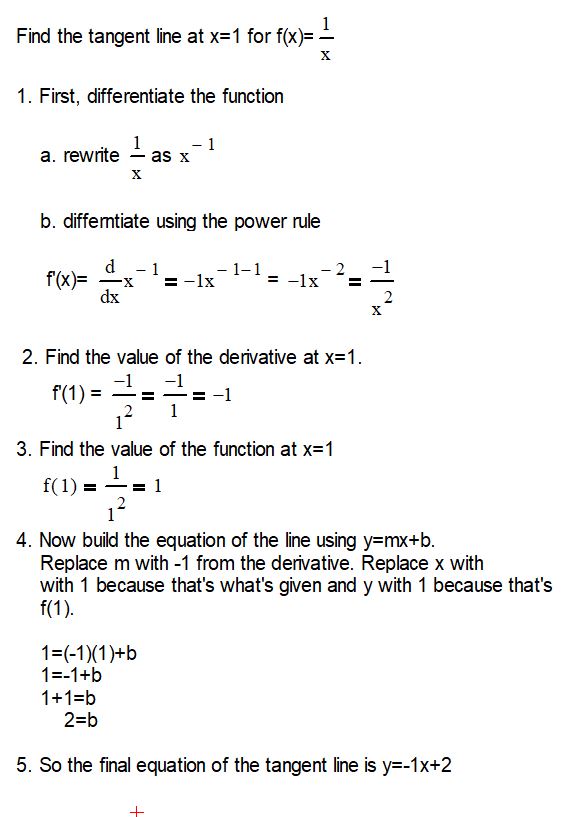
How to Find the Equation of the Tangent Line to y = 1/x at x = 1

Step 1: Define the Original Function

Math: y = 1/x
Explanation: We start with the function y = 1/x. This function describes a hyperbola.

Step 2: Understand the Goal

Math: Goal: Find the equation of the tangent line at x = 1
Explanation: Our objective is to find the equation of the line that just “touches” the curve y = 1/x at the point where x = 1.

Step 3: Differentiate the Function

Math: y’ = -1/x²
Explanation: To find the slope of the tangent line, we first find the derivative of y = 1/x, which is y’ = -1/x².

Step 4: Plug in the Point of Tangency

Math: y'(1) = -1/1² = -1
Explanation: We evaluate the derivative at x = 1 to find the slope of the tangent line at that point. The slope is -1.

Step 5: Use the Point-Slope Equation

Math: y – 1 = -1(x – 1)
Explanation: Using the point-slope equation, y – y₁ = m(x – x₁), where m = -1 and the point (x₁, y₁) is (1, 1), we find the equation of the tangent line to be y – 1 = -1(x – 1).

Step 6: Simplify into Slope-Intercept Form

Math: y – 1 = -1(x – 1)
Explanation: Start with the point-slope form of the equation of the tangent line.

Math: y – 1 = -x + 1
Explanation: Distribute the -1 across (x – 1).

Math: y = -x + 2
Explanation: Add 1 to both sides to isolate y.

Final Equation: y = -x + 2
Explanation: The equation of the tangent line is y = -x + 2, which is in the slope-intercept form y = mx + b.