Mastering Quadratic Equations: From Roots to Polynomials

Reconstructing an Equation from Zeros/Roots

Learn the step-by-step process to rebuild a quadratic equation from its given roots. This guide is ideal for students and educators seeking to understand the fundamentals of algebra.

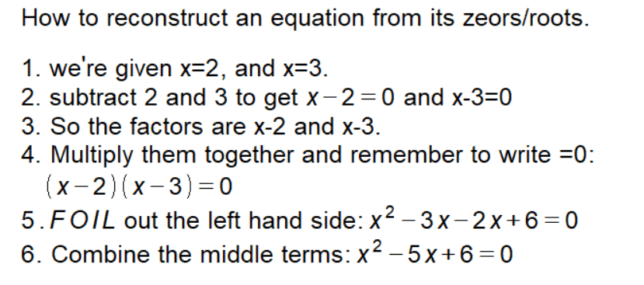
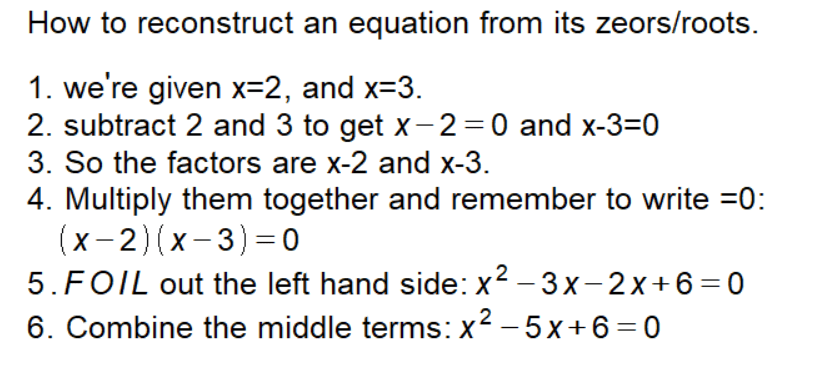
  1. We’re given roots at x = 2, and x = 3.
  2. Subtract the roots from x to get x − 2 = 0 and x − 3 = 0.
  3. The factors derived from the roots are x − 2 and x − 3.
  4. Multiply the factors: (x − 2)(x − 3) = 0.
  5. Apply the FOIL method on the left hand side: x² − 3x − 2x + 6 = 0.
  6. Combine like terms to get the final equation: x² − 5x + 6 = 0.

Understanding the relationship between roots and the quadratic equation enhances problem-solving skills in algebra.

Formulating a Quadratic Equation from Roots

Learn the process of creating a quadratic equation from specific roots. This guide simplifies algebraic concepts for educational purposes.

  1. Given the roots: x = -1 and x = 2.
  2. Convert roots to factors by changing the sign: x + 1 = 0 and x - 2 = 0.
  3. The corresponding factors are: (x + 1) and (x - 2).
  4. Multiply the factors to form the equation: (x + 1)(x - 2) = 0.
  5. Expand using distribution: x² - 2x + x - 2 = 0.
  6. Simplify by combining like terms: x² - x - 2 = 0.

Mastering the technique of forming equations from roots is essential for solving algebraic problems effectively.

Creating a Quadratic Equation from Fractional and Whole Roots

A stepwise guide to derive a quadratic equation from roots, including a fractional root. Ideal for educational insights into quadratic constructions.

  1. Given roots: x = 1/2 and x = 3.
  2. Rewrite the fractional root as 2x = 1 leading to 2x - 1 = 0.
  3. For the whole root, write the factor as x - 3 = 0.
  4. The factors corresponding to the roots are: (2x - 1) and (x - 3).
  5. Multiply these factors to get the equation: (2x - 1)(x - 3) = 0.
  6. Expand the product: 2x² - 6x - x + 3 = 0.
  7. Combine like terms to simplify: 2x² - 7x + 3 = 0.

Understanding how to handle fractional roots is crucial for algebraic proficiency and problem-solving accuracy.

Deriving a Quadratic Equation from Fractional Roots

This tutorial demonstrates the construction of a quadratic equation from roots that are fractions, providing a clear method for students to follow.

  1. Start with the roots: x = -1/2 and x = 2/3.
  2. For the root x = -1/2, multiply by 2 to clear the fraction: 2x = -1, leading to the factor 2x + 1 = 0.
  3. For the root x = 2/3, multiply by 3 to clear the fraction: 3x = 2, which gives the factor 3x - 2 = 0.
  4. The equation is formed by the product of these factors: (2x + 1)(3x - 2) = 0.
  5. Expand the factors: 2x(3x) + 2x(-2) + 1(3x) - 1(2) = 0, which simplifies to 6x² - 4x + 3x - 2 = 0.
  6. Combine the like terms to finalize the equation: 6x² - x - 2 = 0.

Grasping the concept of forming equations from fractional roots is vital for comprehensive algebraic problem-solving.

Constructing a Quadratic Equation from General Fractional Roots

A guide for forming a quadratic equation from roots expressed as fractions. This method is crucial for algebraic skills development.

  1. Given the fractional roots: x = a/b and y = c/d.
  2. Eliminate the fractions by multiplying each root by its denominator to find integer-equivalent expressions: bx = a leading to bx - a = 0, and dy = c leading to dy - c = 0.
  3. The factors corresponding to the roots are: (bx - a) and (dy - c).
  4. The quadratic equation is formed by the product of these factors: (bx - a)(dy - c) = 0.
  5. Expand the product to form the equation: bxdy - bxc - ady + ac = 0.
  6. For a standard quadratic form, divide through by the common denominator bd if necessary, resulting in: x² - (ac + bd)x + ac = 0, after simplifying and collecting like terms.

This general approach is adaptable to any fractional roots and is a foundational technique in algebra.