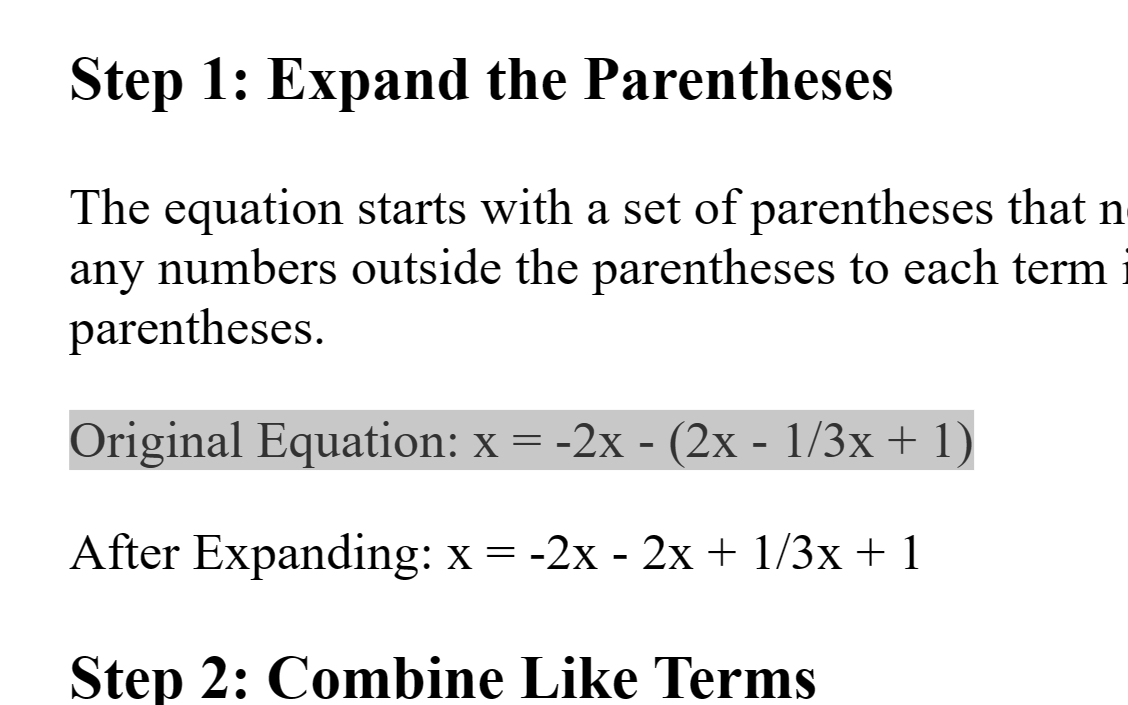
Step 1: Expand the Parentheses
The equation starts with a set of parentheses that need to be expanded. Expanding means to remove the parentheses by distributing any numbers outside the parentheses to each term inside. In this case, we distribute the negative sign to each term inside the parentheses.
Original Equation: x = -2x – (2x – 1/3x + 1)
After Expanding: x = -2x – 2x + 1/3x + 1
Step 2: Combine Like Terms
After expanding, the next step is to combine like terms. ‘Like terms’ are terms that have the same variable raised to the same power. Here, we have several terms with the variable ‘x’. We combine these terms together to simplify the equation.
Combining Like Terms: x = -6x/3 – 6x/3 + x/3 + 1
Simplified Equation: x = -11x/3 – 1
Step 3: Move All Terms to One Side
Now, we want to isolate ‘x’ on one side of the equation to solve for it. To do this, we move all terms involving ‘x’ to one side of the equation and the constant terms to the other side. This sets the stage for solving for ‘x’.
Moving Terms: x + 11x/3 = -1
Combining ‘x’ Terms: 14x/3 = -1
Step 4: Solve for x
Finally, we solve for ‘x’ by getting rid of the fraction. We multiply both sides of the equation by 3 to eliminate the denominator. Then we divide both sides by 14 to isolate ‘x’.
Multiplying by 3: 14x = -3
Dividing by 14: x = -3/14
So the solution to the equation x = -2x – (2x – 1/3x + 1) is x = -3/14.