Solution for x⁴ = 16x²
Step 1: Rewrite the equation as x⁴ – 16x² = 0
Step 2: Factor out the common term x²
x²(x² – 16) = 0
Step 3: Factor the expression x² – 16 as a difference of two squares
x²(x – 4)(x + 4) = 0
Step 4: Solve for x by setting each factor equal to zero
x² = 0 ⟶ x = 0
x – 4 = 0 ⟶ x = 4
x + 4 = 0 ⟶ x = -4
Step 5: The equation x⁴ = 16x² has three solutions: x = 0, x = 4, and x = -4
Solving A Variety Of Equation Types Math 111 Notes 9 18 2023
Solving the Equation x³ – 3x² + 3x – 9 = 0 by Factoring
🔹 Step 1: Group the Terms
Group the terms in pairs: (x³ – 3x²) + (3x – 9)
🔹 Step 2: Factor Each Group
Factor out the common terms from each group:
x²(x – 3) + 3(x – 3)
🔹 Step 3: Factor by Grouping
Now, factor out the common binomial (x – 3):
(x – 3)(x² + 3)
🔹 Step 4: Find the Roots
Set each factor equal to zero and solve for x:
x – 3 = 0 ⟶ x = 3
x² + 3 = 0 ⟶ x = ±√(-3) = ±√3i
🔹 Step 5: Final Answer
The equation x³ – 3x² + 3x – 9 = 0 has roots x = 3, x = √3i, and x = -√3i.
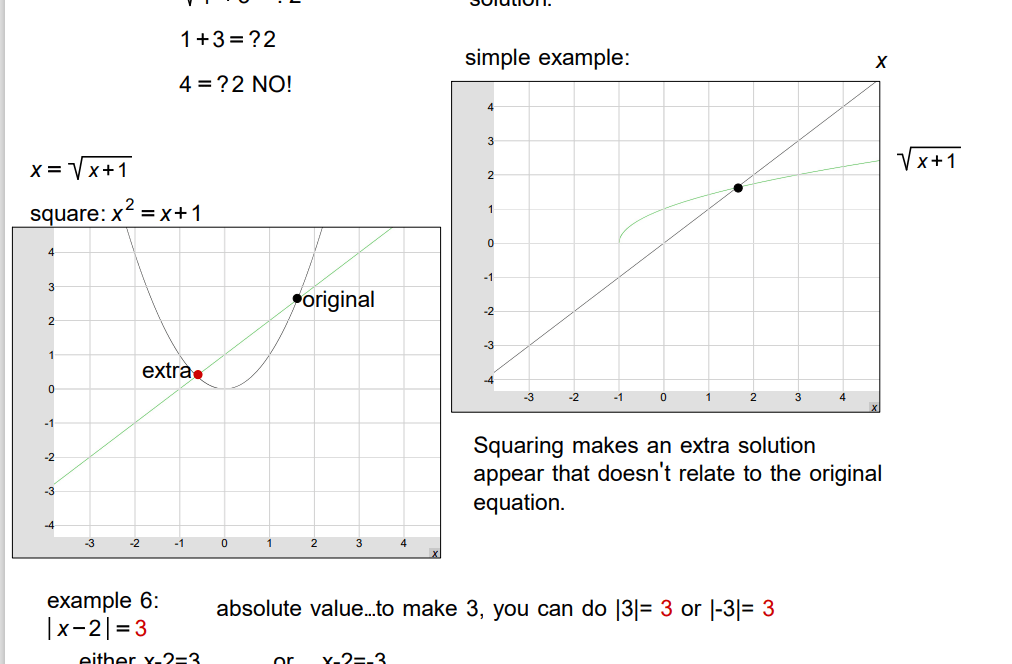
Solving the Equation √(2x + 7) – x = 2
🔹 Step 1: Isolate the Square Root
Move the term with x to the other side of the equation:
√(2x + 7) = x + 2
🔹 Step 2: Square Both Sides
Squaring both sides to eliminate the square root:
(√(2x + 7))² = (x + 2)²
2x + 7 = x² + 4x + 4
🔹 Step 3: Simplify the Equation
Rearrange the equation into standard form:
x² + 2x – 3 = 0
🔹 Step 4: Factor the Equation
Factor the equation:
(x + 3)(x – 1) = 0
🔹 Step 5: Solve for x
Set each factor equal to zero and solve for x:
x + 3 = 0 ⟶ x = -3
x – 1 = 0 ⟶ x = 1
🔹 Step 6: Check the Solutions
Substitute the solutions back into the original equation to check:
For x = -3: √(2(-3) + 7) – (-3) = 2 ⟶ False
For x = 1: √(2(1) + 7) – 1 = 2 ⟶ True
🔹 Step 7: Final Answer
The equation √(2x + 7) – x = 2 has one solution x = 1.