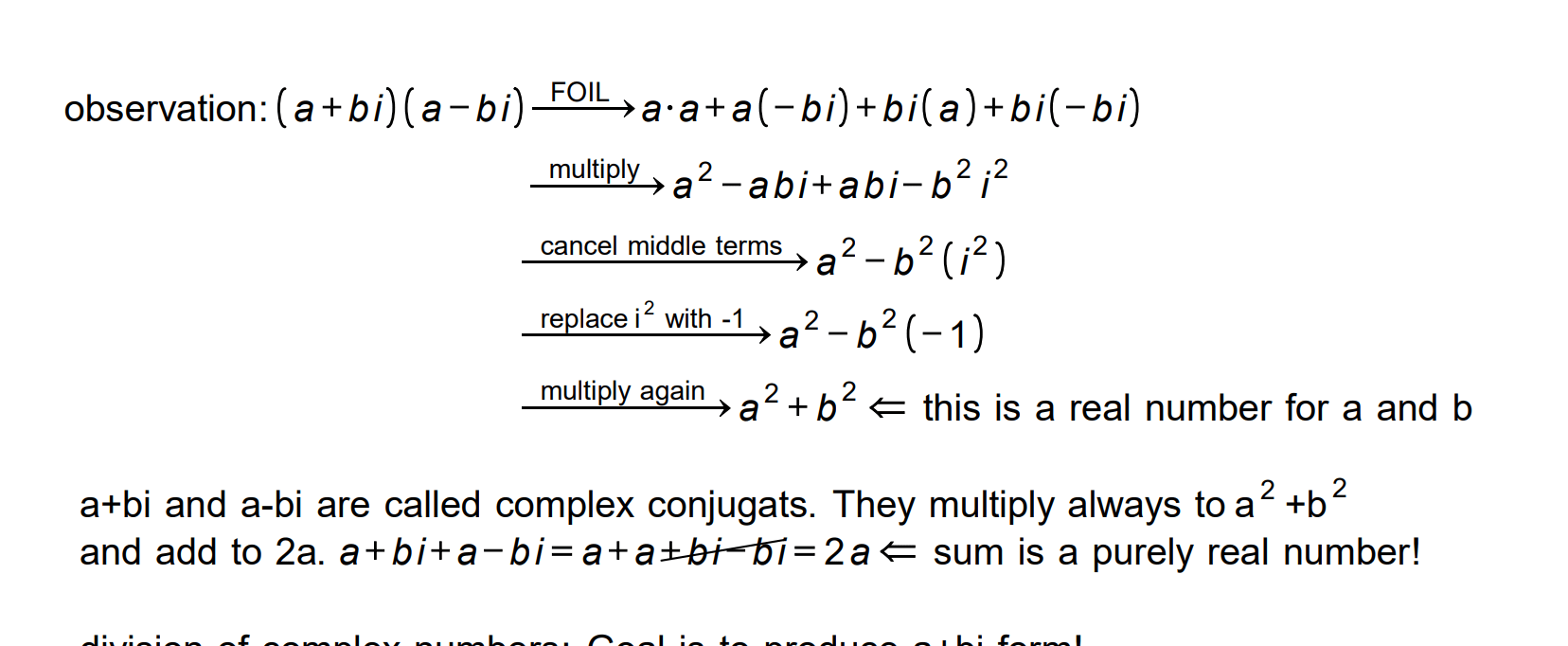
Unlock the mysteries of Complex Numbers with this comprehensive guide, tailored for Math 111, Section 1.5. Delve into the basics with our easy-to-understand explanation of what complex numbers are, consisting of real and imaginary components. Master arithmetic operations such as addition, subtraction, multiplication, and division, all formulated for complex numbers. We break down the distributive and commutative properties for effortless calculations. Learn the ins and outs of polar forms and their applications, all explained with clarity. From electrical engineering to quantum physics, our guide lays the groundwork for understanding the crucial role that complex numbers play in various scientific fields
Applications of Complex Numbers
Physics
- Quantum Mechanics: \( \psi(x) = Ae^{ikx} \)
- Electrodynamics: \( Z = R + iX \)
- Wave Mechanics: \( \Psi(x,t) = Ae^{i(kx – \omega t)} \)
Engineering
- Electrical Engineering: \( V(t) = Ae^{i\omega t} \)
- Control Theory: \( F(s) = \frac{N(s)}{D(s)} \), where \( s = \sigma + i\omega \)
- Mechanical Vibrations: \( x(t) = A\cos(\omega t) + iB\sin(\omega t) \)
- Fluid Dynamics: Navier-Stokes: \( i\omega u – \nu \nabla^2 u = -\nabla p \)
- Telecommunications: \( S(t) = Ae^{i2\pi f t} \)
Computer Science
- Image Processing: \( F(u,v) = \int\int f(x,y)e^{-i2\pi(ux+vy)}dxdy \)
- Machine Learning: Complex-valued neural networks
- Data Compression: \( X(k) = \sum_{n=0}^{N-1} x(n)e^{-i2\pi kn/N} \)
Mathematics
- Calculus: \( \oint_C f(z)dz \)
- Differential Equations: \( ay” + by’ + cy = Ce^{i\omega t} \)
- Number Theory: Gaussian Integers \( a+bi \)
- Linear Algebra: \( Ax = \lambda x \), \( \lambda = a + bi \)
- Optimization: \( z = re^{i\theta} \)
Economics
- Game Theory: Payoff matrices involving \( i \)
- Financial Modeling: \( S(t) = S(0)e^{(r – i\sigma^2)t} \)
Geography
- Seismology: Wave equations with \( i \)
- Oceanography: \( \eta(x,t) = Ae^{i(kx – \omega t)} \)
Medicine
- MRI Technology: \( M(x) = \int\rho(x)e^{-ikx}dx \)
- Biomedical Engineering: \( H(s) = \frac{Y(s)}{X(s)} \) where \( s = \sigma + i\omega \)
Other Applications
- Music: \( f(t) = A_1\sin(2\pi f_1t) + iA_2\sin(2\pi f_2t) \)
- Astronomy: Celestial mechanics involving \( i \)
- Cryptography: \( E(x) = (ax + bi) \mod m \)
- Robotics: \( Z = x + iy \) for control systems