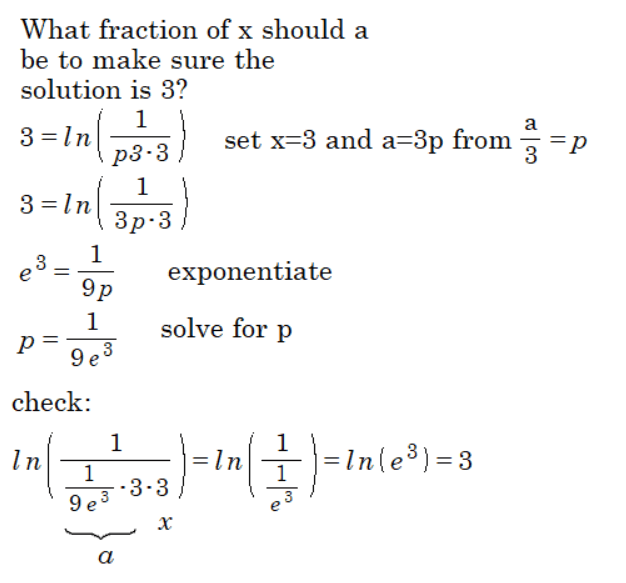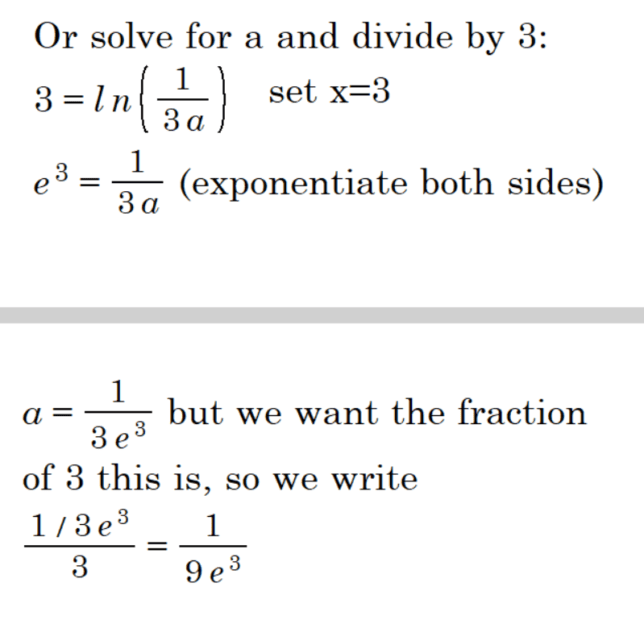What fraction of x should a be to make x equals 3 the solution to x=ln(1/(ax))

To find the fraction of x that a must be to ensure the solution is 3, consider this:

1. Start with x = ln(1/(ax)). Set x = 3 to solve for a.

• Rewrite ln(1/(ax)) in exponential form:
  e^x = 1/(ax).
• Isolate a:
  a = 1/(x * e^x).
• With x = 3, the value for a is:
  a = 1/(3 * e^3).
• To get the fraction that a represents of x:
  fraction = 1/(9 * e^3).

Thus, the fraction of x that a must be to ensure the solution is 3 is 1/(9 * e^3).